ORCCA Quantities in the Physical World Chapter Review

Section 8.5 Quantities in the Physical World Chapter Review

Subsection 8.5.1 Scientific Notation

In Section 1 we covered the definition of scientific notation, how to convert to and from scientific notation, and how to do some calculations in scientific notation.

🔗

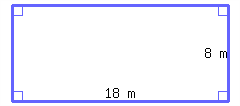
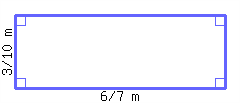
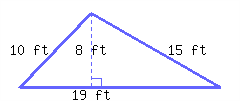
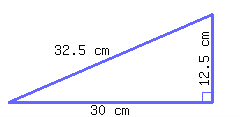
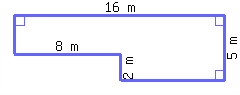
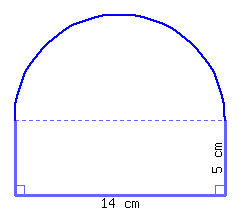
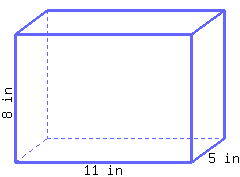
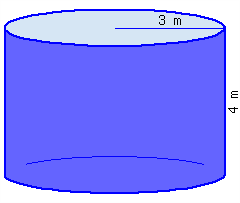
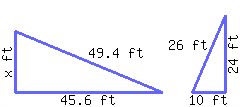
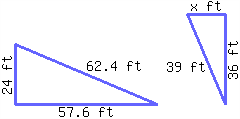
Example 8.5.1. Scientific Notation for Large Numbers.

🔗

The distance to the star Betelgeuse is about $3,780,000,000,000,000$ miles. Write this number in scientific notation.
The gross domestic product (GDP) of California in the year 2017 was about $$ 2.746 \times 10^{13} .$ Write this number in standard notation.

Explanation.

$3,780,000,000,000,000 = 3.78 \times 10^{15} .$
$$ 2.746 \times 10^{13} = $ 2,746,000,000,000 .$

🔗

Example 8.5.2. Scientific Notation for Small Numbers.

🔗

Human DNA forms a double helix with diameter $2 \times 10^{- 9}$ meters. Write this number in standard notation.
A single grain of Forget-me-not (Myosotis) pollen is about $0.00024$ inches in diameter. Write this number in scientific notation.

Explanation.

$2 \times 10^{- 9} = 0.000000002 .$
$0.00024 = 2.4 \times 10^{- 4} .$

🔗

Example 8.5.3. Multiplying and Dividing Using Scientific Notation.

🔗

The fastest spacecraft so far have traveled about

5 \times 10^{6}

miles per day.

If that spacecraft traveled at that same speed for $2 \times 10^{4}$ days (which is about $55$ years), how far would it have gone? Write your answer in scientific notation.
The nearest star to Earth, besides the Sun, is Proxima Centauri, about $2.5 \times 10^{13}$ miles from Earth. How many days would you have to fly in that spacecraft at top speed to reach Proxima Centauri

Explanation.

Remember that you can find the distance traveled by multiplying the rate of travel times the time traveled: $d = r \cdot t .$ So this problem turns into

$\begin{aligned} d & = r \cdot t \\ d & = (5 \times 10^{6}) \cdot (2 \times 10^{4}) \end{aligned}$
Multiply coefficient with coefficient and power of $10$ with power of $10 .$
$\begin{aligned} = (5 \cdot 2) (10^{6} \times 10^{4}) \\ = 10 \times 10^{10} \end{aligned}$
Remember that this still isn’t in scientific notation. So we convert like this:
$\begin{aligned} = 1.0 \times 10^{1} \times 10^{10} \\ = 1.0 \times 10^{11} \end{aligned}$

So, after traveling for $2 \times 10^{4}$ days (55 years), we will have traveled about $1.0 \times 10^{11}$ miles. That’s one-hundred million miles. I hope someone remembered the snacks.
Since we are looking for time, let’s solve the equation $d = r \cdot t$ for $t$ by dividing by $r$ on both sides: $t = \frac{d}{r} .$ So we have:

$\begin{aligned} t & = \frac{d}{r} \\ t & = \frac{2.5 \times 10^{13}}{5 \times 10^{6}} \end{aligned}$
Now we can divide coefficient by coefficient and power of $10$ with power of $10 .$
$\begin{aligned} t & = \frac{2.5}{5} \times \frac{10^{13}}{10^{6}} \\ t & = 0.5 \times 10^{7} \\ t & = 5 \times 10^{- 1} \times 10^{7} \\ t & = 5 \times 10^{6} \end{aligned}$

This means that to get to Proxima Centauri, even in our fastest spacecraft, would take $5 \times 10^{6}$ years. Converting to standard form, this is $5,000,000$ years. I think we’re going to need a faster ship.

🔗

Subsection 8.5.2 Unit Conversion

🔗

Unit conversion is a particular process that uses unit ratios to convert units. You may refer to Appendix A to find unit conversion facts needed to do these conversions.

🔗

Example 8.5.4. Using Multiple Unit Ratios.

🔗

How many grams are in

5

pounds?

\begin{aligned} 5 lb & = \frac{5 lb}{1} & Rewrite as a ratio. \\ = \frac{5 lb}{1} \cdot \frac{1 kg}{2.205 lb} \cdot \frac{1000 g}{1 kg} & Two unit ratios are needed. \\ = \frac{5 lb}{1} \cdot \frac{1 kg}{2.205 lb} \cdot \frac{1000 g}{1 kg} & Units may now cancel. \\ = \frac{5}{1} \cdot \frac{1}{2.205} \cdot \frac{1000 g}{1} & Only units of g remain. \\ = \frac{5 \cdot 1000}{2.205} g & Multiply what's left and then divide. \\ \approx 2268 g \end{aligned}

🔗

5

pounds is about

2268

grams.

🔗

Example 8.5.5. Converting Squared or Cubed Units.

🔗

Convert

240

square inches into square centimeters.

\begin{aligned} 240 {in}^{2} & = \frac{240 {in}^{2}}{1} & Rewrite as a ratio. \\ = \frac{240 {in}^{2}}{1} \cdot {(\frac{2.54 cm}{1 in})}^{2} & The unit ratio needs to be squared. \\ = \frac{240 {in}^{2}}{1} \cdot \frac{{2.54}^{2} {cm}^{2}}{1 {in}^{2}} & Everything inside the parentheses is squared. \\ = \frac{240 {in}^{2}}{1} \cdot \frac{{2.54}^{2} {cm}^{2}}{1 {in}^{2}} & Units may now cancel. \\ = \frac{240}{1} \cdot \frac{{2.54}^{2} {cm}^{2}}{1} & Only units of sq cm remain. \\ = 240 \cdot {2.54}^{2} {cm}^{2} & Multiply. \\ \approx 1548 {cm}^{2} \end{aligned}

🔗

240

square inches is approximately

1548

square centimeters.

🔗

Example 8.5.6. Converting Rates.

🔗

Gold has a density of 19.3 ^g⁄_mL. What is this density in ounces per cubic inch?

\begin{aligned} 19.3 \frac{g}{mL} & = \frac{19.3 g}{1 mL} & Write the rate as a ratio. \\ \approx \frac{19.3 g}{1 mL} \cdot \frac{16.39 mL}{1 {in}^{3}} \cdot \frac{1 oz}{28.35 g} & Use unit ratios to make cancellations. \\ = \frac{19.3 g}{1 mL} \cdot \frac{16.39 mL}{1 {in}^{3}} \cdot \frac{1 oz}{28.35 g} & Units may now cancel. \\ = \frac{19.3}{1} \cdot \frac{16.39}{1 {in}^{3}} \cdot \frac{1 oz}{28.35} & Only oz per cubic inch remain. \\ = \frac{19.3 \cdot 16.39}{28.35} \frac{oz}{{in}^{3}} & Multiply what's left and then divide. \\ \approx 11.16 \frac{oz}{{in}^{3}} \end{aligned}

🔗

Notice that we did not need to raise any unit ratios to a power since there is a conversion fact that tells us that

1 {in}^{3} \approx 16.39 mL .

🔗

Thus, the density of gold is about 11.16 ^oz⁄_in³.

🔗

Subsection 8.5.3 Geometry Formulas

🔗

In Section 3 we established the following formulas.

Perimeter of a Rectangle: 🔗
$P = 2 (ℓ + w)$
Area of a Rectangle: 🔗
$A = ℓ w$
Area of a Triangle: 🔗
$A = \frac{1}{2} b h$
Circumference of a Circle: 🔗
$c = 2 π r$
Area of a Circle: 🔗
$A = π r^{2}$
Volume of a Rectangular Prism: 🔗
$V = w d h$
Volume of a Cylinder: 🔗
$V = π r^{2} h$
Volume of a Rectangular Prism or Cylinder: 🔗
$V = B h$

🔗

Write the following number in scientific notation.

🔗

11000 =

🔗

2.

🔗

Write the following number in scientific notation.

🔗

210 =

🔗

3.

🔗

Write the following number in scientific notation.

🔗

0.031 =

🔗

4.

🔗

Write the following number in scientific notation.

🔗

0.0041 =

🔗

Exercise Group.

🔗

5.

🔗

Write the following number in decimal notation without using exponents.

🔗

5.09 \times 10^{2} =

🔗

6.

🔗

Write the following number in decimal notation without using exponents.

🔗

6.09 \times 10^{5} =

🔗

7.

🔗

Write the following number in decimal notation without using exponents.

🔗

7.09 \times 10^{0} =

🔗

8.

🔗

Write the following number in decimal notation without using exponents.

🔗

8.08 \times 10^{0} =

🔗

9.

🔗

Write the following number in decimal notation without using exponents.

🔗

9.07 \times 10^{- 2} =

🔗

10.

🔗

Write the following number in decimal notation without using exponents.

🔗

1.08 \times 10^{- 3} =

🔗

Exercise Group.

🔗

11.

🔗

Multiply the following numbers, writing your answer in scientific notation.

🔗

(2 \times 10^{3}) (2 \times 10^{5}) =

🔗

12.

🔗

Multiply the following numbers, writing your answer in scientific notation.

🔗

(3 \times 10^{5}) (7 \times 10^{4}) =

🔗

Exercise Group.

🔗

13.

🔗

Divide the following numbers, writing your answer in scientific notation.

🔗

\frac{1.6 \times 10^{3}}{4 \times 10^{- 4}} =

🔗

14.

🔗

Divide the following numbers, writing your answer in scientific notation.

🔗

\frac{1 \times 10^{4}}{5 \times 10^{- 5}} =

🔗

Unit Conversion.

🔗

15.

🔗

Convert

2.48 q t

to pints.

🔗

16.

🔗

Convert

616 p t

to cups.

🔗

17.

🔗

Convert

9.93 o z

to pounds.

🔗

18.

🔗

Convert

46.1 k m

to feet.

🔗

19.

🔗

Convert

87 w k

to hours.

🔗

20.

🔗

Convert

3.5 m s

to nanoseconds.

🔗

21.

🔗

Convert

2.8 d m^{3}

to cubic meters.

🔗

22.

🔗

Convert

97 f t^{2}

to square yards.

🔗

23.

🔗

Convert

5.6 \frac{d a m}{w k}

to meters per day.

🔗

24.

🔗

Convert

91.4 \frac{m^{2}}{d}

to hectares per hour.

🔗

25.

🔗

Convert

391 \frac{m g}{d}

to grams per hour.

🔗

26.

🔗

Convert

7.68 \frac{M B}{h}

to gigabytes per minute.

🔗

Exercise Group.

🔗

27.

🔗

Chris’s bedroom has

188 f t^{2}

of floor. He would like to carpet the floor, but carpeting is sold by the square yard. How many square yards of carpeting will he need to get?

🔗

28.

🔗

Lindsay is traveling in Europe and renting a car. She is used to thinking of gasoline amounts in gallons, but in Europe it is sold in liters. After filling the gas tank, she notices it took

26 L

of gas. How many gallons is that?

🔗

29.

🔗

Will was driving at a steady speed of

26 m p h

for

19

minutes. How far did he travel in that time?

🔗

30.

🔗

The algae in a pond is growing at a rate of

0.19 \frac{k g}{d} .

How much algae is in the pond after

12

weeks?

🔗

Geometry.

🔗

31.

🔗

Find the perimeter and area of the rectangle.

🔗

Its perimeter is and its area is .

🔗

32.

🔗

Find the perimeter and area of the rectangle.

🔗

Its perimeter is and its area is .

🔗

Exercise Group.

🔗

33.

🔗

Find the area of the rectangle below.

🔗

34.

🔗

Find the perimeter and area of a rectangular table top with a length of

5.3 f t

and a width of

24 i n .

🔗

Its perimeter is and its area is .

🔗

35.

🔗

Find the perimeter and area of the triangle.

🔗

Its perimeter is and its area is .

🔗

36.

🔗

Find the perimeter and area of the right triangle.

🔗

Its perimeter is and its area is .

🔗

37.

🔗

The area of the triangle below is square feet.

🔗

38.

🔗

Find the area of a triangular flag with a base of

2.2 m

and a height of

120 c m .

🔗

Its area is .

🔗

39.

🔗

Find the perimeter and area of this shape.

🔗

Its perimeter is and its area is .

🔗

40.

🔗

Find the perimeter and area of this polygon.

🔗

Its perimeter is and its area is .

🔗

Exercise Group.

🔗

41.

🔗

The formula

A = \frac{1}{2} r n s

gives the area of a regular polygon with side length

s,

number of sides

n

and, apothem

r .

(The apothem is the distance from the center of the polygon to one of its sides.)

🔗

What is the area of a regular pentagon with

s = 45 i n

and

r = 98 i n ?

🔗

42.

🔗

A circle’s radius is

6 m .

🔗

The circumference, in terms of $π,$ is .
This circle’s circumference, rounded to the hundredths place, is .
This circle’s area, in terms of $π,$ is .
This circle’s area, rounded to the hundredths place, is .

🔗

Exercise Group.

🔗

43.

🔗

Find the perimeter and area of this shape, which is a semicircle on top of a rectangle.

🔗

Its perimeter is and its area is .

🔗

44.

🔗

Find the volume of this rectangular prism.

🔗

45.

🔗

A cube’s side length is

9 c m .

Its volume is .

🔗

46.

🔗

Find the volume of this cylinder.

🔗

This cylinder’s volume, in terms of $π,$ is .
This cylinder’s volume, rounded to the hundredths place, is .

🔗

47.

🔗

A cylinder’s base’s diameter is

12 f t,

and its height is

2 f t .

🔗

This cylinder’s volume, in terms of $π,$ is .
This cylinder’s volume, rounded to the hundredths place, is .

🔗

48.

🔗

A cylinder’s base’s diameter is

6 f t,

and its height is

3 f t .

🔗

This cylinder’s volume, in terms of $π,$ is .
This cylinder’s volume, rounded to the hundredths place, is .

🔗

49.

🔗

Fill out the table with various formulas as they were given in this section.

Rectangle Perimeter
Rectangle Area
Triangle Area
Circle Circumference
Circle Area
Rectangular Prism Volume
Cylinder Volume
Volume of either Rectangular Prism or Cylinder