Skip to main content
Logo image

Intermediate Algebra: Functions and Graphs

Katherine Yoshiwara

Section A.10 Chapter 10 Logarithmic Functions

Subsection A.10.1 Logarithmic Functions

Subsubsection A.10.1.1 Graph log functions

One way to graph a log function is to first make a table of values for its inverse function, the exponential function with the same base, then interchange the variables.
🔗
Checkpoint A.10.1.
  1. Complete the table of values and graph on the same grid:  f(x)=x3  and  g(x)=3x 
       x       0       1       2       3       4       5       6       8      10   
     f(x) 
     g(x) 
    Grid for 3 to x and x-cubed
  2. Use your tables from part (a) to graph  h(x)=x3  and  j(x)=log3⁡(x)  on the same grid.
    Grid for log base 3 and cube root
🔗
Answer.
  1. 3 to x and x-cubed
  2. log base 3 and cube root
🔗
🔗

Subsubsection A.10.1.2 Use function notation

A log function is the inverse of the exponential function with the same base, and vice versa.
🔗
Example A.10.2.
For each function  f(x) , decide whether f(a+b)=f(a)+f(b).
🔗
  1. f(x)=3x
  2. f(x)=log3⁡(x)
🔗
Solution.
  1. f(a+b)=3a+b,  and  f(a)+f(b)=3a+3b. 
    But  3a+b  is not equivalent to  3a+3b;  in fact  3a+b=3a⋅3b.
    So for this function, f(a+b)≠f(a)+f(b).
  2. f(a+b)=log3⁡(a+b),  and  f(a)+f(b)=log3⁡(a)+log3⁡(b). 
    But  log3⁡(a+b)  is not equivalent to  log3⁡(a)+log3⁡9;  in fact  log3⁡(ab)=log3⁡(a)+log3⁡(b).
    So for this function, f(a+b)≠f(a)+f(b).
🔗
🔗
Checkpoint A.10.3.
g(x)=5x . Evaluate and simplify if possible.
🔗
  1. g(3+t)
  2. g(3t)
🔗
Answer.
  1. 125⋅5t
  2. 125t
🔗
Example A.10.4.
q(x)=9x  and p(x) is its inverse function. Evaluate if possible.
🔗
  1. q(12)
  2. p(3)
  3. q(0)
  4. p(0)
🔗
Solution.
  1. 3
  2. 12
  3. 1
  4. undefined
🔗
🔗
Checkpoint A.10.5.
h(x)=log4⁡(x).  Evaluate if possible.
🔗
  1. h(4)
  2. g(4), where g is the inverse function for h
  3. h(0)
  4. g(0)
🔗
Answer.
  1. 1
  2. 256
  3. undefined
  4. 1
🔗
Checkpoint A.10.6.
f(x)=log8⁡(x) . Evaluate and simplify if possible.
🔗
  1. f(64p)
  2. f(64+p)
🔗
Answer.
  1. 2+log8⁡(p)
  2. cannot be simplified
🔗
🔗

Subsubsection A.10.1.3 Use the properties of logarithms

The three properties of logarithms are helpful in making computations involving logs.
🔗
Properties of Logarithms.
If x, y, b>0, and b≠1, then
🔗
  1. logb⁡(xy)=logb⁡(x)+logb⁡(y)
  2. logb⁡(xy)=logb⁡(x)−logb⁡(y)
  3. logb⁡(xk)=klogb⁡(x)
🔗
🔗
Example A.10.7.
If logb⁡(10)=2.303 and logb⁡(2)=0.693, what is logb⁡(5)?
🔗
Solution.
Because 5=102,
🔗
logb⁡(5)=logb⁡(102)=logb⁡(10)−logb⁡(2)=2.303−0.693=1.61
🔗
🔗
Example A.10.8.
If logb⁡(10)=2.303 and logb⁡(2)=0.693, what is logb⁡(20)?
🔗
Solution.
Because 20=10⋅2,
🔗
logb⁡(20)=logb⁡(10⋅2)=logb⁡(10)+logb⁡(2)=2.303+0.693=2.996
🔗
🔗
Checkpoint A.10.9.
Take the log of each number. What do you notice?
🔗
  1. 8⋅100=800
  2. 12⋅1000=12,000
  3. 20⋅25=500
  4. 200⋅250=50,000
🔗
Answer.
  1. log⁡(8)+log⁡(10)=log⁡(800)
  2. log⁡(12)+log⁡(100)=log⁡(12,000)
  3. log⁡(20)+log⁡(25)=log⁡(500)
  4. log⁡(200)+log⁡(250)=log⁡(50,000)
🔗
Checkpoint A.10.10.
Compare the two operations. What do you notice?
🔗
  1. (i) Compute 102.68                  (ii) Solve for x:  log⁡(x)=2.68
  2. (i) Compute 10−0.75                 (ii) Solve for x:  log⁡(x)=−0.75
🔗
Answer.
  1. (i) and (ii) have the same answer: 478.63
  2. (i) and (ii) have the same answer: 0.1778
🔗
Checkpoint A.10.11.
  1. The ratio of N to P is 32.6. Compute log⁡(N)−log⁡(P).
  2. log⁡(z)−log⁡(t)=2.5. Compute zt.
🔗
Answer.
  1. 1.5132
  2. 316.2278
🔗
🔗
🔗

Subsection A.10.2 Logarithmic Scales

Subsubsection A.10.2.1 Plot a log scale

Because log⁡(x) grows very slowly, we can use logs to compare quantities that vary greatly in magnitude.
🔗
Example A.10.12.
  1. Complete the table. Round the values to one decimal place.
    x  1   5  25 125 625
    log⁡(x)
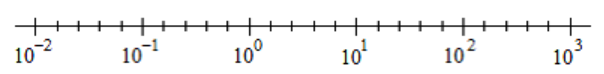
  2. Plot the values of x on a log scale.
    log scale
  3. Each time we multiply x by 5, how much does the logarithm increase? What is log⁡(5), to one decimal place?
🔗
Solution.
  1. x  1   5  25 125 625
    log⁡x 0 0.7 1.4 2.1 2.8
  2. log scale
  3. Each time we multiply x by 5, the log of x increases by 0.7, because log⁡(5)=0.7. This is an application of the log properties:
    log⁡(5x)=log⁡(x)+log⁡(5)=log⁡(x)+0.7
🔗
🔗
Checkpoint A.10.13.
  1. Complete the table. Round the values to one decimal place.
    x  5   10   20   40   80 
    log⁡(x)
  2. Plot the values of x on a log scale.
    log scale
  3. Each time we multiply x by 2, how much does the logarithm increase? What is log⁡(2), to one decimal place?
🔗
Answer.
  1. x  5   10   20   40   80 
    log⁡(x) 0.7 1 1.3 1.6 1.9
  2. log scale
  3. 0.3; 0.3
🔗
Checkpoint A.10.14.
  1. Complete the table. Round the values to one decimal place.
    x 0.25  1   4   16   64  256
    log⁡(x)
  2. Plot the values of x on a log scale.
    log scale
  3. Each time we multiply x by 4, how much does the logarithm increase? What is log⁡(4), to one decimal place?
🔗
Answer.
  1. x 0.25  1   4   16   64  256
    log⁡(x) −0.6 0 0.6 1.2 1.8 2.4
  2. log scale
  3. 0.6; 0.6
🔗
🔗

Subsubsection A.10.2.2 Compare quantities

There is often more than one way to express a comparison with mathematical notation.
🔗
Example A.10.15.
When we say that "A is 3 times larger than B," we mean that A=3B.
🔗
🔗
Example A.10.16.
When we say that "A is 3 more than B," we mean that A=B+3.
🔗
🔗
Use these equations for the following Checkpoints.
🔗
  1. x=5H
  2. x=5H
  3. x=5+H
  4. H=x+5
  5. H=5x
  6. H=5x
  7. x−H=5
  8. H−x=5
  9. xH=5
  10. Hx=5
  11. log⁡xlog⁡H=5
  12. log⁡x−log⁡H=log⁡5
  13. log⁡x+log⁡5=log⁡H
🔗
Checkpoint A.10.17.
From the list above, match all the correct algebraic expressions to the phrase "x is 5 times as large as H."
🔗
Answer.
(a), (i), (l)
🔗
Checkpoint A.10.18.
From the list above, match all the correct algebraic expressions to the phrase "x is 5 more than H."
🔗
Answer.
(c), (g)
🔗
🔗
🔗

Subsection A.10.3 The Natural Base

Subsubsection A.10.3.1 Graphs of y=ex and y=ln⁡(x)

The graphs of the natural exponential function and the natural log function have some special properties.
🔗
Checkpoint A.10.19.
Use technology to graph  f(x)=ex  and  y=x+1  in a window with  −2≤(x)≤3  and  −1≤y≤4 . What do you notice about the two graphs?
🔗
Answer.
graph
The line is tangent to the graph at (0,1).
🔗
Checkpoint A.10.20.
Use technology to graph  f(x)=ln⁡x  and  y=x−1  in a window with  −1≤(x)≤4  and  −2≤y≤3 . What do you notice about the two graphs?
🔗
Answer.
graph
The line is tangent to the graph at (1,0).
🔗
🔗

Subsubsection A.10.3.2 Using growth and decay laws with base e

We can write exponential growth and decay laws using base e.
🔗
Exponential Growth and Decay.
The function
🔗
P(t)=P0ekt
describes exponential growth if k>0, and exponential decay if k<0.
🔗
🔗
🔗
Example A.10.21.
A colony of bees grows at a rate of 8% annually. Write its growth law using base e.
🔗
Solution.
The growth factor is  b=1+r=1.08 , so the growth law can be written as
🔗
P(t)=P0(1.08)t
Using base e, we write  P(t)=P0ekt,  where ek=1.08. (You can see this by evaluating each growth law at t=1.) So we solve for k.
🔗
ek=1.08Take the natural log of both sides.ln⁡(ek)=ln⁡(1.08)Simplify both sides.k=0.0770
The growth law is  P(t)=P0e0.077t.
🔗
🔗
🔗
Example A.10.22.
A radioactive isotope decays according to the formula  N(t)=N0e−0.016t,  where t is in hours. Find its percent rate of decay.
🔗
Solution.
First we write the decay law in the form  N(t)=N0bt,  where  b=ek. 
🔗
In this case,  k=−0.016,  so  b=e−0.016=0.9841.  Now,  b=1−r,  and solving for r we find  r=−0.0159.  The rate of decay is approximately 16% per hour.
🔗
🔗
Checkpoint A.10.23.
A virus spreads in the population at a rate of 19.5% daily. Write its growth law using base e.
🔗
Answer.
P(t)=P0e0.178t
🔗
Checkpoint A.10.24.
Sea ice is decreasing at a rate of 12.85% per decade. Write its decay law using base e.
🔗
Answer.
Q(t)=Q0e−0.1375t
🔗
Checkpoint A.10.25.
In 2020, the world population was growing according to the formula  P(t)=P0e0.0488t,  where t is in years. Find its percent rate of growth.
🔗
Answer.
5%
🔗
Checkpoint A.10.26.
Since 1984, the population of cod has decreased annually according to the formula  N(t)=N0e−0.1863t.  Find its percent rate of decay.
🔗
Answer.
17%
🔗
🔗
🔗
You have attempted 1 of 1 activities on this page.
🔗
PrevTopNext