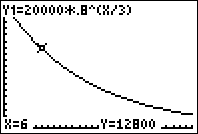Skip to main content
Logo image

Intermediate Algebra: Functions and Graphs

Katherine Yoshiwara

Appendix C Answers to Selected Exercises

1 Linear Models
1.1 Creating a Linear Model
1.1.3 Problem Set 1.1

Warm Up

1.1.3.1.
Answer.
  1. 5.5
  2. 12
1.1.3.3.
Answer.
I=200+0.09S

Skills Practice

1.1.3.5.
Answer.
65
1.1.3.7.
Answer.
y>65
1.1.3.9.
Answer.
R=50−0.4w

Applications

1.1.3.11.
Answer.
  1. t (days) 0 5 10 15 20
    h (inches) 6 16 26 36 46
  2. h=6+2t
  3. grid
  4. 48 in
  5. 33 days
  6. h=6+2(21); 72=6+2t
  7. 14 is the initial height of the plants in inches. 1.5 is the number of inches they grow each day.
1.1.3.13.
Answer.
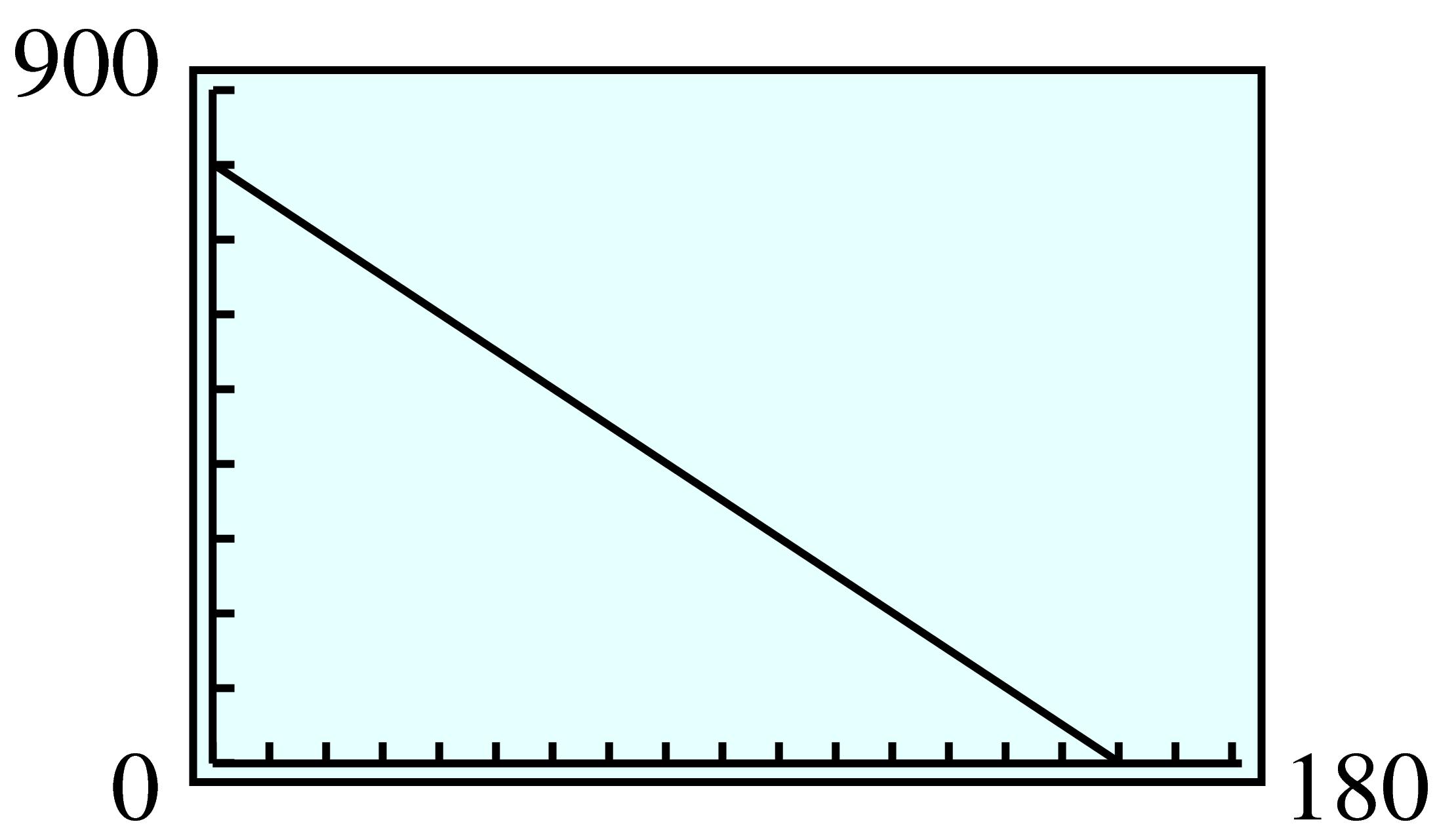
  1. Altitude (1000 ft) 0 1 2 3 4 5
    Boiling point (∘F) 212 210 208 206 204 202
  2. boiling point
  3. 4∘F
  4. Over 4000 feet

1.2 Graphs and Equations
1.2.6 Problem Set 1.2

Warm Up

1.2.6.1.
Answer.
  1. no
  2. yes
  3. no
  4. no
1.2.6.3.
Answer.
  1. yes
  2. no
  3. no
  4. yes
1.2.6.5.
Answer.
  1. no
  2. no
  3. yes
  4. yes
1.2.6.7.
Answer.
horizontal: 0.25; vertical: 4
1.2.6.9.
Answer.
  1. horizontal: 5; vertical: 250
  2. points on grid

Skills Practice

1.2.6.11.
Answer.
−154
1.2.6.13.
Answer.
35
1.2.6.17.
Answer.
x≥72
number line
1.2.6.19.
Answer.
y=2x3+24
1.2.6.21.
Answer.
y=−713x+7
1.2.6.23.
Answer.
y=29x+17

Applications

1.2.6.25.
Answer.
    1. x=−6
    2. x<−6
    3. x>−6
    1. x=−6
    2. x<−6
    3. x>−6
1.2.6.27.
Answer.
  1. x=0.3
  2. x=0.8
  3. x≤0.3
  4. x≥0.8
1.2.6.29.
Answer.
  1. x≤4
  2. x>2
1.2.6.31.
Answer.
  1. x=4
  2. x<22
1.2.6.33.
Answer.
  1. p=120+5t
  2. increasing blood pressure
  3. 141
  4. 7.5 min

1.3 Intercepts
1.3.6 Problem Set 1.3

Warm Up

1.3.6.1.
Answer.
  1. 5x
  2. 2y
  3. 5x+2y=1000
1.3.6.3.
Answer.
y=−35x+165

Skills Practice

1.3.6.5.
Answer.
  1. (4,0), (0,−3)
  2. line with positive intercepts
1.3.6.7.
Answer.
  1. (−8,0), (0,5)
  2. line with positive intercepts
1.3.6.9.
Answer.
  1. (3,0),(0,5)
  2. (12,0),(0,−14)
  3. (52,0),(0,−32)
  4. (p,0),(0,q)
  5. The value of a is the x-intercept, and the value of b is the y-intercept.
1.3.6.11.
Answer.
  1. (0,b)
  2. (−bm,0)
1.3.6.13.
Answer.
−2x+3y=2400
1.3.6.15.
Answer.
3x+400y=240
1.3.6.17.
Answer.
  1. 22x+9y=33, and
  2. y=113+−229x
1.3.6.19.
Answer.
  1. 4x+12y=48, and
  2. y=4−13x

Applications

1.3.6.21.
Answer.
  1. 9x
  2. 4y
  3. 9x+4y=1800
  4. (200,0) Delbert must eat 200g of figs daily if he eats no bananas.
  5. (0,450) Delbert must eat 450g of bananas daily if he eats no figs.
1.3.6.23.
Answer.
  1.  t  0 5 10 15 20
     h  −400 −300 −200 −100 0
  2. h=−44+20t
  3. grid
  4. (0,−400): The diver starts at a depth of 400 feet. (20,0): The diver surfaces after 20 minutes.
1.3.6.25.
Answer.
  1. $2.40x, $3.20y
  2. 2.40x+3.20y=19,200
  3. line
  4. The y-intercept, 6000 gallons, is the amount of premium that the gas station owner can buy if he buys no regular. The x-intercept, 8000 gallons, is the amount of regular he can buy if he buys no premium.

1.4 Slope
1.4.5 Problem Set 1.4

Warm Up

1.4.5.1.
Answer.
Anthony
1.4.5.3.
Answer.
Bob’s driveway
1.4.5.5.
Answer.
−1
1.4.5.7.
Answer.
−23

Skills Practice

1.4.5.9.
Answer.
  1. 32
  2. 6, 32
  3. −9, 32
  4. 27
1.4.5.11.
Answer.
34
1.4.5.13.
Answer.
−4000
1.4.5.15.
Answer.
  1. 9x+12y=36
  2. m=−34
1.4.5.17.
Answer.
14.29 ft
1.4.5.19.
Answer.
(a)

Applications

1.4.5.21.
Answer.
  1. del x and del y
  2. 800
  3. P=7000+800t
1.4.5.23.
Answer.
  1. −6 liters/day
  2. The water supply is decreasing at a rate of 6 liters per day.
  3. line with changes in W and t
  4. W=48−6t
1.4.5.25.
Answer.
  1. Yes, the slope is 0.12
  2. 0.12 cm/kg: The spring stretches an extra 0.12 cm for each additional 1 kg mass.
1.4.5.27.
Answer.
  1. The distances to the stations are known.
  2. 5.7 km/sec
1.4.5.29.
Answer.
  1. 7.9
  2. 8.35 km
  3. 2 hrs 5 min

1.5 Equations of Lines
1.5.6 Problem Set 1.5

Warm Up

1.5.6.1.
Answer.
x −1  0   2   3   4 
y 10 8 4 2 0
line
  1. 8
  2. decreases by 2 units
  3. The constant term is the y-intercept and the coefficient of x is the slope.
1.5.6.3.
Answer.
  1. Δh=22
  2. Δr=−57

Skills Practice

1.5.6.5.
Answer.
  1. y=−32x+12
  2. m=−32; b=12
1.5.6.7.
Answer.
  1. y=14x−22
  2. m=14; b=−22
1.5.6.9.
Answer.
  1. line
  2. y=−53x−6
  3. x=−185
1.5.6.11.
Answer.
  1. II
  2. III
  3. I
  4. IV
1.5.6.13.
Answer.
  1. line through (2,-5) with slope -3
  2. y+5=−3(x−2)
  3. y=−3x+1
1.5.6.15.
Answer.
y=4x+40
1.5.6.17.
Answer.
  1. III
  2. IV
  3. II
  4. I

Applications

1.5.6.19.
Answer.
  1. M=7000−400w
  2. The slope tells us that Tammy’s bank account is diminishing at a rate of $400 per week, the vertical intercept that she had $7000 (when she lost all sources of income).
1.5.6.21.
Answer.
m=−0.0018 degree/foot, so the boiling point drops with altitude at a rate of 0.0018 degree per foot. b=212, so the boiling point is 212∘ at sea level (where the elevation h=0).
1.5.6.23.
Answer.
  1. h 8 20
    C 645 1425
  2. C=125+65h
  3. m=65, the lesson rate is 65 dollars per hour
1.5.6.25.
Answer.
  1. C 15 −5
    F 59 23
  2. F=32+95C
  3. m=95, so an increase of 1∘C is equivalent to an increase of 95∘F.
1.5.6.27.
Answer.
  1. 55∘F
  2. 9840 ft
  3. temperature vs altitude
  4. m=−3820. The temperature decreases 3 degrees for each increase in altitude of 820 feet.
  5. (19,13313,0). At an altitude of 19,13313 feet, the temperature is 0∘F. (0,70). At an altitude of 0 feet, the temperature is 70∘F.
1.5.6.29.
Answer.
  1. (0,28)
    salt dissolved
  2. m=12
  3. y=12x+28
  4. 36∘C

1.6 Chapter Summary and Review
1.6.3 Chapter 1 Review Problems

1.6.3.1.

Answer.
  1. n 100 500 800 1200 1500
    C 4000 12,000 18,000 26,000 32,000
  2. C=20n+2000
  3. cost of producing calculators
  4. $22,000
  5. 400

1.6.3.2.

Answer.
  1. t  5   10   15   20   25 
    R 1960 1820 1680 1540 1400
  2. R=2100−28t
  3. (75,0), (0,2100)
    grid
  4. t-intercept: The oil reserves will be gone in 2080; R-intercept: There were 2100 billion barrels of oil reserves in 2005.

1.6.3.3.

Answer.
  1. 60C+100T=1200
  2. (20,0), (0,12)
    grid
  3. 6 days
  4. She can spend 20 days in Atlantic City if she spends no time in Saint-Tropez, or 12 days in Saint-Tropez if she spends no time in Atlantic City.

1.6.3.4.

Answer.
line with x-intercept 5, y-intercept -12

1.6.3.5.

Answer.
line with x-intercept -400, y-intercept 500

1.6.3.6.

Answer.
line with x-intercept 6, y-intercept 4

1.6.3.7.

Answer.
line through origin with slope 3/4

1.6.3.8.

Answer.
line through origin with slope -1/4

1.6.3.9.

Answer.
verticalline x= -3

1.6.3.10.

Answer.
  1. B=800−5t
  2. GC graph
  3. m=−5 thousand barrels/minute: The amount of oil in the tanker is decreasing by 5000 barrels per minute.

1.6.3.11.

Answer.
  1. F=500+0.10C
  2. GC graph
  3. m=0.10: The fee increases by $0.10 for each dollar increase in the remodeling job.

1.6.3.12.

Answer.
−32

1.6.3.13.

Answer.
2

1.6.3.14.

Answer.
−0.4

1.6.3.15.

Answer.
−1.75

1.6.3.16.

Answer.
neither

1.6.3.17.

Answer.
both

1.6.3.18.

Answer.
d V
−5 −4.8
−2 −3
1 −1.2
6 1.8
10 4.2

1.6.3.19.

Answer.
q S
−8 −8
−4 36
3 168
5 200
9 264

1.6.3.20.

Answer.
80 ft

1.6.3.21.

Answer.
m=12, b=−54

1.6.3.22.

Answer.
m=34, b=54

1.6.3.23.

Answer.
m=−4, b=3

1.6.3.24.

Answer.
m=0, b=3

1.6.3.25.

Answer.
  1. line through (-4,6), slope -2/3
  2. y=−23x+103

1.6.3.26.

Answer.
  1. line through (2,-5), slope 3/2
  2. y=32x−8

1.6.3.27.

Answer.
  1. T=62−0.0036h
  2. −46∘F; 108∘F
  3. −71∘F

1.6.3.28.

Answer.
y=−95x+25

1.6.3.29.

Answer.
y=−52x+8

1.6.3.30.

Answer.
  1. t 0 15
    P 4800 6780
  2. P=4800+132t
  3. m=132 people/year: the population grew at a rate of 132 people per year.

1.6.3.31.

Answer.
  1. m=−2; b=3
  2. y=−2x+3

1.6.3.32.

Answer.
  1. m=32; b=−5
  2. y=32x−5

1.6.3.33.

Answer.
35

1.6.3.34.

Answer.
  1. (4,0) and (0,−6)
  2. 32

1.6.3.35.

Answer.
  1. (83,0) and (0,−4)
  2. (4,3); No

2 Applications of Linear Models
2.1 Linear Regression
2.1.5 Problem Set 2.1

Warm Up

2.1.5.1.
Answer.
a: II; b: III; c: I; d: IV
2.1.5.2.
Answer.
a: III; b: IV; c: II; d: I
2.1.5.3.
Answer.
  1. k 70 50
    p 154 110
  2. p=2.2k
  3. m=2.2 pounds/kg is the conversion factor from kilograms to pounds

Skills Practice

2.1.5.5.
Answer.
The slope is −2.5, which indicates that the snack bar sells 2.5 fewer cups of cocoa for each 1∘C increase in temperature. The C-intercept of 52 indicates that 52 cups of cocoa would be sold at a temperature of 0∘C. The T-intercept of 20.8 indicates that no cocoa will be sold at a temperature of 20.8∘C.
2.1.5.7.
Answer.
scatterplot and line
2.1.5.9.
Answer.
  1. L=24+(29/7)t, where t is in months
  2. 74 feet
  3. The young whale grows in length about 4.14 foot per month
2.1.5.11.
Answer.
2 min: 21∘C; 2 hr: 729∘C; The estimate at 2 minutes is reasonable; the estimate at 2 hours is not reasonable.

Applications

2.1.5.13.
Answer.
  1. 12 seconds
  2. 39
  3. scatterplot with regression line
  4. 11.6 seconds
  5. y=8.5+0.1x
  6. 12.7 seconds; 10.18 seconds; The prediction for the 40-year-old is reasonable, but not the prediction for the 12-year-old.
2.1.5.15.
Answer.
  1. graph
  2. 53 sec, 64 sec
  3. y=5.5x−8.6
  4. 57.95 sec
2.1.5.17.
Answer.
  1. scatterplot with regression line
  2. y=121+19.86t
  3. 419
2.1.5.19.
Answer.
  1. scatterplot and regression line
  2. The graph is above.
  3. The slope tells us that the time it takes for a bird to attract a mate decreases by 0.85 days for every additional song it learns.
  4. 44.5 days
  5. The C-intercept tells us that a warbler with a repretoire of 53 songs would acquire a mate immediately. The B-intercept tells us that a warbler with no songs would take 62 days to find a mate. These values make sense in context.

2.2 Linear Systems
2.2.5 Problem Set 2.2

Warm Up

2.2.5.1.
Answer.
line
2.2.5.3.
Answer.
18.75

Skills Practice

2.2.5.5.
Answer.
(−4,5)
2.2.5.7.
Answer.
(−2,3)
2.2.5.9.
Answer.
Inconsistent
2.2.5.11.
Answer.
Dependent

Applications

2.2.5.13.
Answer.
  1. Sporthaus: y=500+10x
    Fitness First: y=50+25x
  2. x Sporthaus Fitness First
    6 560 200
    12 620 350
    18 680 500
    24 740 650
    30 800 800
    36 860 950
    42 920 1100
    48 980 1250
  3. Cost for fitness clubs
  4. 30 months
2.2.5.15.
Answer.
  1. 10x+5y=300
  2. y=3x
  3. two lines
    (12,36)
  4. She should buy 12 hardbacks and 36 paperbacks.
2.2.5.17.
Answer.
  1. S=1.5x
  2. D=120−2.5x
  3. two lines
  4. x=30; S=45
2.2.5.19.
Answer.
  1. The median age
  2. grid
  3. 0.3 years of age per year since 1990
  4. See (b) above
  5. Slightly less than 14 years since 1990
  6. More than half the women are older than the mean age of women.

2.3 Algebraic Solution of Systems
2.3.5 Problem Set 2.3

Warm Up

2.3.5.1.
Answer.
(4,1)

Skills Practice

2.3.5.3.
Answer.
(1,2)
2.3.5.7.
Answer.
(1,2)
2.3.5.9.
Answer.
Dependent

Applications

2.3.5.11.
Answer.
  1. S=2.5x;  D=350−4.5x
  2. 50 dollars per machine; 125 machines
2.3.5.13.
Answer.
  1. Pounds % silver Amount of silver
    First alloy x 0.45 0.45x
    Second alloy y 0.60 0.60y
    Mixture 40 0.48 0.48(40)
  2. x+y=40
  3. 0.45x+0.60y=19.2
  4. 32 lb
2.3.5.15.
Answer.
  1. Rani’s speed in still water: x
    Speed of the current: y
    Rate Time Distance
    Downstream x+y 45 6000
    Upstream x−y 45 4800
  2. 45(x+y)=6000
  3. 45(x−y)=4800
  4. Rani’s speed in still water is 120 meters per minute, and the speed of the current is 1313 meters per minute.
2.3.5.17.
Answer.
607.5 mi
2.3.5.19.
Answer.
  1. Sports coupes Wagons Total
    Hours of riveting 3 4 120
    Hours of welding 4 5 155
  2. 3x+4y=120
  3. 4x+5y=155
  4. 20 sports coupes and 15 wagons

2.4 Gaussian Reduction
2.4.6 Problem Set 2.4

Warm Up

2.4.6.1.
Answer.
(−3,−5)
2.4.6.3.
Answer.
  1. Principal Interest rate Interest
    Bonds x 0.10 0.10x
    Certificate y 0.08 0.08y
    Total 2000 —— 184
  2. x+y=2000
  3. 0.10x+0.08y=184

Skills Practice

2.4.6.5.
Answer.
(−2,0,3)
2.4.6.7.
Answer.
(2,−2,0)
2.4.6.9.
Answer.
(2,−3,1)
2.4.6.11.
Answer.
(12,23,−3)
2.4.6.13.
Answer.
(12,−12,13)
2.4.6.15.
Answer.
Dependent

Applications

2.4.6.17.
Answer.
x=40 in, y=60 in, z=55 in
2.4.6.19.
Answer.
12 lb Colombian, 14 lb French, 14 Sumatran
2.4.6.21.
Answer.

2.5 Linear Inequalities in Two Variables
2.5.5 Problem Set 2.5

Warm Up

2.5.5.1.
Answer.
  1. x −2 5 6 8
    y 12 5 4 2
  2. grid
  3. x+y=10
  4. See above.
2.5.5.3.
Answer.
  1. The graph of the equation is a line, and the graph of the inequality is a half-plane. The line is the boundary of the half-plane but is not included in the solution to the inequality.
  2. The graph of x+y≥10,000 includes both the line x+y=10,000 and the half-plane of the corresponding strict inequality.

Skills Practice

2.5.5.5.
Answer.
linear inequality in two variables
2.5.5.7.
Answer.
linear inequality in two variables
2.5.5.9.
Answer.
linear inequality in two variables
2.5.5.11.
Answer.
linear inequality in two variables
2.5.5.13.
Answer.
linear inequality in two variables
2.5.5.15.
Answer.
system of inequalities
2.5.5.17.
Answer.
system of inequalities with vertices
2.5.5.19.
Answer.
system of inequalities
2.5.5.21.
Answer.
system of inequalities with vertices
2.5.5.23.
Answer.
system of inequalities, with vertices

Applications

2.5.5.25.
Answer.
system of inequalities
2.5.5.27.
Answer.
graph

2.6 Chapter Summary and Review
2.6.3 Chapter 2 Review Problems

2.6.3.1.

Answer.
  1. regression line for boiling point vs heat of vaporization
  2. 235 kilojoules
  3. y=0.106x+4.6
  4. 156.7∘C

2.6.3.2.

Answer.
scatterplot with regression line
  1. 45 cm
  2. 87 cm
  3. y=1.2x−3
  4. 69 cm
  5. y=1.197x−3.660; 68.16 cm

2.6.3.3.

Answer.
6

2.6.3.4.

Answer.
26 min

2.6.3.5.

Answer.
(−1,2)

2.6.3.6.

Answer.
(1.9,−0.8)

2.6.3.7.

Answer.
(12,72)

2.6.3.8.

Answer.
(1,2)

2.6.3.9.

Answer.
(1,2)

2.6.3.10.

Answer.
(12,32)

2.6.3.11.

Answer.
Consistent and independent

2.6.3.12.

Answer.
Inconsistent

2.6.3.13.

Answer.
Dependent

2.6.3.14.

Answer.
Consistent and independent

2.6.3.15.

Answer.
(2,0,−1)

2.6.3.16.

Answer.
(2,1,−1)

2.6.3.17.

Answer.
(2,−5,3)

2.6.3.18.

Answer.
(2,32,−1)

2.6.3.19.

Answer.
(−2,1,3)

2.6.3.20.

Answer.
(2,−1,0)

2.6.3.21.

Answer.
26

2.6.3.22.

Answer.
17

2.6.3.23.

Answer.
$3181.82 at 8%, $1818.18 at 13.5%

2.6.3.24.

Answer.
$4000

2.6.3.25.

Answer.
5 cm, 12 cm, 13 cm

2.6.3.26.

Answer.
20 to Boston, 25 to Chicago, 10 to Los Angeles

2.6.3.27.

Answer.
strict inequality

2.6.3.28.

Answer.
strict inequality

2.6.3.29.

Answer.
strict inequality

2.6.3.30.

Answer.
compound inequality

2.6.3.31.

Answer.
system of inequalities

2.6.3.32.

Answer.
system of inequalities

2.6.3.33.

Answer.
system of inequalities

2.6.3.34.

Answer.
system of inequalities

2.6.3.35.

Answer.
system of inequalities

2.6.3.36.

Answer.
system of inequalities

2.6.3.37.

Answer.
system of inequalities

2.6.3.38.

Answer.
system of inequalities

2.6.3.39.

Answer.
20p+9g≤120, 10p+10g≤120, p≥0, g≥0
system of inequalities

2.6.3.40.

Answer.
x+y≤32, 2x+1.6y≥56, x≥0, y≥0, where x represents ounces of tofu, y the ounces of tempeh
system of inequalities

3 Quadratic Models
3.1 Extraction of Roots
3.1.9 Problem Set 3.1

Warm Up

3.1.9.1.
Answer.
  1. −12
  2. −2
  3. 9
3.1.9.3.
Answer.
  1. 29
  2. 7
  3. 6
3.1.9.4.
Answer.
  1. ±7
  2. ±52
  3. ±5
3.1.9.5.
Answer.
  1. parabola
  2. Two solutions. ≈±2.5
  3. ±6

Skills Practice

3.1.9.7.
Answer.
±3
3.1.9.9.
Answer.
52, −32
3.1.9.11.
Answer.
23±53
3.1.9.13.
Answer.
78,±88
3.1.9.15.
Answer.
±5.73
3.1.9.17.
Answer.
b. 10, -2
3.1.9.19.
Answer.
±Frm
3.1.9.21.
Answer.
±piL8

Applications

3.1.9.23.
Answer.
21 in
3.1.9.25.
Answer.
  1. A=1600(1+r)2
  2.  r  0.02 0.04 0.06 0.08
     A  1664.64 1730.56 1797.76 1866.24
  3. 11.8%
3.1.9.27.
Answer.
19 ft by 57 ft
3.1.9.29.
Answer.
  1. V=62.8r2
  2. 14
  3. 1.96 cm
3.1.9.31.
Answer.
  1. ±bca
  2. ±acb

3.2 Intercepts, Solutions, and Factors
3.2.6 Problem Set 3.2

Warm Up

3.2.6.1.
Answer.
  1. 2b2+9b−18
  2. 12z2−35z+8
3.2.6.3.
Answer.
  1. 6p3−33p2+45p
  2. 6v3+16v2−32v
3.2.6.5.
Answer.
  1. (x−4)(x+4)
  2. x(x−16)
  3. (x−4)(x−4)
  4. cannot be factored
3.2.6.7.
Answer.
(x−5)(x−2)
3.2.6.9.
Answer.
(w−8)(w+4)
3.2.6.11.
Answer.
(x−5)(x−2)

Skills Practice

3.2.6.13.
Answer.
12, −3
3.2.6.15.
Answer.
0, 3
3.2.6.17.
Answer.
12, 1
3.2.6.19.
Answer.
−1,2
3.2.6.21.
Answer.
78±88
3.2.6.23.
Answer.
b±5a
3.2.6.25.
Answer.
0.1(x−18)(x+15)
3.2.6.27.
Answer.
All three graphs have the same x-intercepts.

Applications

3.2.6.29.
Answer.
c. 306.5 ft at 0.625 sec d. 1.25 sec e. 5 sec
3.2.6.31.
Answer.
b. h2+102=(h+2)2 c. 24 ft
3.2.6.33.
Answer.
  1. l=x−4, w=x−4, h=2, V=2(x−4)2
  2. 0 cubic in, 2 cubic in, 8 cubic in, etc.
  3. x=4
  4. 9 in by 9 in
  5. 2(x−4)2=50; x=9
3.2.6.34.
Answer.
a. l=6, w=1−2x, h=x, V=6x(1−2x)   e. 6x(1−2x)=34;  14  ft

3.3 Graphing Parabolas
3.3.6 Problem Set 3.3

Warm Up

3.3.6.1.
Answer.
13
3.3.6.4.
Answer.
  1. factoring; 0,10
  2. extraction of roots; ±10
  3. factoring; −12,1
  4. extraction of roots; −52,−52
3.3.6.6.
Answer.
2πR(R+H)
3.3.6.8.
Answer.
0,−35

Skills Practice

3.3.6.9.
Answer.
  1. ii
  2. iv
  3. i
  4. iii
  5. vi
  6. v
3.3.6.11.
Answer.
    1. Narrower
    2. graph y=2x-squared
    1. Wider
    2. graph y=2x-squared
    1. Opens downward
    2. graph y=neg x-squared
3.3.6.13.
Answer.
(0,0),(4,0);(2,−4)
parabola
3.3.6.15.
Answer.
(0,0),(−2,0);(−1,−3)
parabola
3.3.6.17.
Answer.
(0,0),(20,0);(10,200)
parabola

Applications

3.3.6.19.
Answer.
  1. (2000,400)
  2. (0,0),(4000,0)
  3. parabola
  4. x>4000
3.3.6.21.
Answer.
  1. basic parabola
  2. narrower
  3. wider
  4. narrower and reflected about y-axis
3.3.6.23.
Answer.
  1. x-intercepts at 0 and 4
  2. x-intercepts at 0 and −4
  3. reflection of (a) about y-axis
  4. reflection of (b) about y-axis

3.4 Completing the Square
3.4.4 Problem Set 3.4

Warm Up

3.4.4.1.
Answer.
−4,52
3.4.4.3.
Answer.
  1. x2+10x+25
  2. x2−12x+36
  3. x2−24x+144
  4. x2+30x+225

Skills Practice

3.4.4.5.
Answer.
b and c
3.4.4.7.
Answer.
−4,−5
3.4.4.9.
Answer.
−1±52
3.4.4.11.
Answer.
−52±454
3.4.4.13.
Answer.
−5,8
3.4.4.15.
Answer.
−3,43

Applications

3.4.4.17.
Answer.
  1. (14±1315,0)
  2. (14,−314)
3.4.4.19.
Answer.
  1. (−2,0), (25,0)
  2. (−45,−715)
3.4.4.21.
Answer.
  1. L2+(L−4)2=202
  2. 12 in by 16 in
3.4.4.23.
Answer.
  1. A=12(x2−y2)
  2. A=12(x−y)(x+y)
  3. 18 sq ft
3.4.4.25.
Answer.
  1. A=(x+y)2
  2. A=x2+2xy+y2
  3. graph
3.4.4.27.
Answer.
−1±1−c
3.4.4.29.
Answer.
b2±b2−4c4
3.4.4.31.
Answer.
±V2w−s2
3.4.4.33.
Answer.
(−15,0),(15,0);(0,255)
3.4.4.35.
Answer.
−3x±32

3.5 Chapter 3 Summary and Review
3.5.3 Chapter 3 Review Problems

3.5.3.1.

Answer.
±2

3.5.3.2.

Answer.
±40

3.5.3.3.

Answer.
−4±20

3.5.3.4.

Answer.
1±157

3.5.3.5.

Answer.
1, 4

3.5.3.6.

Answer.
11.5, 23.5

3.5.3.7.

Answer.
−32, 2

3.5.3.8.

Answer.
2, 2

3.5.3.9.

Answer.
−2, 3

3.5.3.10.

Answer.
−1, 1

3.5.3.11.

Answer.
4x2−29x−24=0

3.5.3.12.

Answer.
9x2−30x+25=0

3.5.3.13.

Answer.
y=(x−3)(x+2.4)

3.5.3.14.

Answer.
y=−(x+1.3)(x−2)

3.5.3.15.

Answer.
  1. Vertex: (0,0), y-int: (0,0), x-int: (0,0)
  2. parabola

3.5.3.17.

Answer.
  1. Vertex: (92,−814), y-int: (0,0), x-int: (0,0), (9,0)
  2. parabola

3.5.3.19.

Answer.
2±10

3.5.3.20.

Answer.
−32±214

3.5.3.21.

Answer.
32±34

3.5.3.22.

Answer.
13±109

3.5.3.23.

Answer.
v=±2Km

3.5.3.24.

Answer.
b=±c2−a2

3.5.3.25.

Answer.
s=±3Vh

3.5.3.26.

Answer.
r=±AP−1

3.5.3.27.

Answer.
9

3.5.3.28.

Answer.
13

3.5.3.29.

Answer.
11%

3.5.3.30.

Answer.
8.5%

3.5.3.31.

Answer.
108≈10.4 in

3.5.3.32.

Answer.
17 in

3.5.3.33.

Answer.
1 sec

3.5.3.34.

Answer.
50 ft by 150 ft

3.5.3.35.

Answer.
A1=x2−(12y2+12y2)=x2−y2;  A2=(x+y)(x−y)=x2−y2

3.5.3.36.

Answer.
A1=π(x+y)2−πx2−πy2=2πxy;  A2=πy(2x)=2πxy

4 Applications of Quadratic Models
4.1 Quadratic Formula
4.1.6 Problem Set 4.1

Warm Up

4.1.6.1.
Answer.
  1. 8−220
  2. 9
4.1.6.3.
Answer.
  1. A=lw, P=2l+2w
    1. A=24 sq ft, P=20 ft
    2. A=24 sq ft, P=22 ft

Skills Practice

4.1.6.5.
Answer.
0.618, -1.618
4.1.6.7.
Answer.
0.232, 1.434
4.1.6.9.
Answer.
1.270, 2.480
4.1.6.11.
Answer.
  1. graph
  2. Approximately (1.5,0) and (−0.3,0)
  3. 32 and −13. These are the x-intercepts of the graph.
4.1.6.13.
Answer.
  1. (5,0) and (1,0); (3,0); no x-intercepts
  2. 1 and 5; 3; 6±i122. The real-valued solutions are the x-intercepts of the graph. If the solutions are complex, the graph has no x-intercepts.
4.1.6.15.
Answer.
  1. x2−4x−1=0
  2. x2−8x+25=0
4.1.6.17.
Answer.
−4±16−64h32
4.1.6.19.
Answer.
v±v2−2asa
4.1.6.21.
Answer.
−1±1+8S2

Applications

4.1.6.23.
Answer.
c. 31.77 mph
4.1.6.25.
Answer.
  1. 357 km
  2. 7100 m
4.1.6.27.
Answer.
b. 10h(2h−6)=2160   c. 12 ft by 18 ft by 10ft
Note 4.1.19.
According to the latest research and data, there has been an increase in the number of tigers, and now the total number of wild tigers worldwide is 5,574, according to the World Animal Foundation at https://worldanimalfoundation.org/advocate/animal-captivity-statistics/

4.2 The Vertex
4.2.5 Problem Set 4.2

Warm Up

4.2.5.1.
Answer.
  1. t 0 0.25 0.5 0.75 1 0.25 1.5
    h −12 −5 0 3 4 3 0
  2. grid
  3. (1,4)
  4. The wrench reaches its greatest height of 4 feet.
  5. The wrench reaches its greatest height 1 second after Francine throws it.
4.2.5.3.
Answer.
  1. y=x2−3x−4
  2. y = -x^2+3x+4

Skills Practice

4.2.5.5.
Answer.
(−2,3)
parabola
4.2.5.7.
Answer.
  1. (3,0)
  2. (3,0)
  3. (3,4)
4.2.5.9.
Answer.
  1. (3,4)
  2. parabola
  3. y=2x2−12x+22
4.2.5.11.
Answer.
  1. y=a(x+2)2+6
  2. 3
4.2.5.13.
Answer.
y=(3x+1)2−5
4.2.5.15.
Answer.
  1. IV
  2. V
  3. I
  4. VII

Applications

4.2.5.17.
Answer.
  1. l=40−w; A=40w−w2
  2. 400 sq yd; 20 yd by 20 yd
4.2.5.19.
Answer.
  1. (table)
  2. 20+2x, 60−3x, (20+2x)(60−3x)
  3. (table)
  4. x=20
  5. graph
  6. $24, $36
  7. $1350, $30, 45 rooms
4.2.5.21.
Answer.
  1. h=−140(x−80)2+164
  2. 160.99 ft
4.2.5.23.
Answer.
5 g; 4 min
4.2.5.25.
Answer.
  1. graph
  2. (−4,0), (0,2)
  3.      x<−4 −4<x<2 x>2
    Y1 − + +
    Y2 − − +
    Y3 + − +

4.3 Curve Fitting
4.3.5 Problem Set 4.3

Warm Up

4.3.5.1.
Answer.
(4,10), (0,−22), (4±5,0)
4.3.5.3.
Answer.
  1. 25−2x
  2. 30+4x
4.3.5.5.
Answer.
y=−x2−2x+8
4.3.5.7.
Answer.
y=−x2

Skills Practice

4.3.5.9.
Answer.
(−2,3,−4)
4.3.5.11.
Answer.
(1,−4,7)
4.3.5.13.
Answer.
a=3, b=1, c=−2

Applications

4.3.5.15.
Answer.
  1. y=a(x−5)2−10=−0.2x2+11x−78
  2. 42
4.3.5.17.
Answer.
D=12n2−32n
4.3.5.19.
Answer.
  1. N=−0.59t2+7.33t−2.54
  2. 2000; 7
4.3.5.21.
Answer.
  1. (0,0.14)
  2. y=−1.786x2+0.14

4.4 Quadratic Inequalities
4.4.6 Problem Set 4.4

Warm Up

4.4.6.1.
Answer.
  1. (−4,0), (6,0)
  2. up
4.4.6.3.
Answer.
  1. (3±12,0)
  2. down
4.4.6.5.
Answer.
  1. [0,4)
  2. (5,8)

Skills Practice

4.4.6.7.
Answer.
  1. grid
  2. See graph.
  3. x>3 or x<−1
4.4.6.9.
Answer.
  1. −12,5
  2. x<−12 or x>15
4.4.6.11.
Answer.
  1. (−∞,−3)∪(6,∞)
  2. (−3,6)
  3. [−2,5]
  4. (−∞,2]∪[5,∞)
4.4.6.13.
Answer.
x<−2 or x>3
4.4.6.15.
Answer.
0≤k≤4
4.4.6.17.
Answer.
(−6,−3)
4.4.6.19.
Answer.
(−∞,−12)∪(4,∞)
4.4.6.21.
Answer.
(−∞,−2.24)∪(2.24,∞)
4.4.6.23.
Answer.
All m
4.4.6.25.
Answer.
−4.8<x<6.2

Applications

4.4.6.27.
Answer.
(−∞,−4.2)∪(2.6,∞)
4.4.6.29.
Answer.
4\tt<16 sec
4.4.6.31.
Answer.
$5<p<$7
4.4.6.33.
Answer.
  1. 60−x;  12+12x
  2. y=(60−x)(12+12x)
  3. 882 bu; 18 trees
  4. Between 10 and 26, inclusive

4.5 Chapter 4 Summary and Review
4.5.3 Chapter 4 Review Problems

4.5.3.1.

Answer.
1, 2

4.5.3.2.

Answer.
3±52

4.5.3.3.

Answer.
−4±82

4.5.3.4.

Answer.
−2±284

4.5.3.5.

Answer.
6±36−12h6

4.5.3.6.

Answer.
3±9+8D2

4.5.3.7.

Answer.
one repeated real solution

4.5.3.8.

Answer.
two complex soutions

4.5.3.9.

Answer.
two complex soutions

4.5.3.10.

Answer.
two distinct real soutions

4.5.3.11.

Answer.
  1. h=100t−2.8t2
  2. (graph)
  3. 893 ft
  4. 1557 sec and 20 sec

4.5.3.12.

Answer.
  1. 1.5 sec, 11.025 m
  2. 7.056 m
  3. 0.6 sec
  4. 0.5 sec, 2.5 sec
  5. (0,0), (3,0). She leaves the springboard at t=0 seconds and returns to the springboard at t=3 seconds.

4.5.3.13.

Answer.
  1. (12,−494), (−3,0), (4,0), (0,−12)
  2. parabola

4.5.3.14.

Answer.
  1. (14,−318), no x-intercepts, (0,−4)
  2. parabola

4.5.3.15.

Answer.
  1. (1,5), (−1.24,0), (3.24,0), (0,4)
  2. parabola

4.5.3.16.

Answer.
  1. (32,74),  no x-intercepts, (0,4)
  2. parabola

4.5.3.17.

Answer.
y=0.2(x−15)2−6

4.5.3.18.

Answer.
  1. (1,5)
  2. y=2x2+4x+7

4.5.3.19.

Answer.
  1. 45
  2. $810

4.5.3.20.

Answer.
  1. R=1200x−80x2
  2. $7.50
  3. $4500

4.5.3.21.

Answer.
  1. y=60(4+2x)(32−4x)
  2. 2

4.5.3.22.

Answer.
  1. R=(20+x)(500−10x)
  2. $35

4.5.3.23.

Answer.
a=1, b=−1, c=−6

4.5.3.24.

Answer.
y=−12x2−4x+10

4.5.3.25.

Answer.
  1. h=36.98t+5.17
  2. 116.1 m, 153.1 m
  3. (graph)
  4. h=−4.858t2+47.67t+0.89
  5. 100.2 m, 113.9 m
  6. (graph)
  7. quadratic

4.5.3.26.

Answer.
  1. y=−0.05x2−0.003x+234.2
  2. (−0.03,234.2)  The velocity of the debris at its maximum height of 234.2 feet. The velocity there is actually zero.

4.5.3.27.

Answer.
(−∞,−2)∪(3,∞)

4.5.3.28.

Answer.
[−3,4]

4.5.3.29.

Answer.
[−1,32]

4.5.3.30.

Answer.
(−∞,−13)∪(2,∞)

4.5.3.31.

Answer.
[−2,2]

4.5.3.32.

Answer.
(−∞,−3)∪(3,∞)

4.5.3.33.

Answer.
  1. R=p(220−14p)
  2. 400<p<480

4.5.3.34.

Answer.
  1. R=p(30−12p)
  2. 20<p<40

5 Functions and Their Graphs
5.1 Functions
5.1.7 Problem Set 5.1

Warm Up

5.1.7.1.
Answer.
−24
5.1.7.3.
Answer.
20
5.1.7.5.
Answer.
−3,12
5.1.7.7.
Answer.
143

Skills Practice

5.1.7.9.
Answer.
  1. input: v, output: x
  2. 15
  3. −1,52.
5.1.7.11.
Answer.
  1. −13
  2. −49
  3. −34
  4. −0.530

Applications

5.1.7.13.
Answer.
(b), (c), (e), and (f)
5.1.7.15.
Answer.
  1. 60
  2. 37.5
  3. 20
5.1.7.17.
Answer.
  1. h(1)=2
  2. 1 month
  3. 1 month
  4. 4000
5.1.7.19.
Answer.
  1. Approximately $1920
  2. $5 or $15
  3. f(12)≈1920;  f(5)=1500, f(15)=1500
  4. 7<d<13
5.1.7.21.
Answer.
  1. F(1992)=7.5%
  2. F(2000)=4%
  3. F(1998+=4.5%, F(2001)=4.5%
5.1.7.25.
Answer.
  1. N(6000)=2000: 2000 cars will be sold at a price of $6000.
  2. decrease
  3. 30,000. At a price of $30,000, they will sell 400 cars.

5.2 Graphs of Functions
5.2.6 Problem Set 5.2

Warm Up

5.2.6.1.
Answer.
y = 3x-4
5.2.6.3.
Answer.
y = 3x-4
5.2.6.5.
Answer.
  1. x>0.6
    y = 1.4x - 0.64
  2. x<−0.4
    y = 1.4x - 0.64

Skills Practice

5.2.6.7.
Answer.
graph
5.2.6.9.
Answer.
graph

Applications

5.2.6.11.
Answer.
  1. −2,0,5
  2. 2
  3. h(−2)=0, h(1)=0, h(0)=−2
  4. 5
  5. 3
  6. increasing: (−4,−2) and (0,3); decreasing: (−2,0)
5.2.6.13.
Answer.
  1. 0, 12, 0
  2. 56
  3. −56, −16, 76, 116
  4. 1, −1
  5. Max at x=−1.5, 0.5, min at x=−0.5, 1.5
5.2.6.15.
Answer.
  1. f(1000)=1495: The speed of sound at a depth of 1000 meters is approximately 1495 meters per second.
  2. d=570 or d=1070: The speed of sound is 1500 meters per second at both a depth of 570 meters and a depth of 1070 meters.
  3. The slowest speed occurs at a depth of about 810 meters and the speed is about 1487 meters per second, so f(810)=1487.
  4. f increases from about 1533 to 1541 in the first 110 meters of depth, then drops to about 1487 at 810 meters, then rises again, passing 1553 at a depth of about 1600 meters.
5.2.6.17.
Answer.
(a) and (d)
5.2.6.19.
Answer.
  1. −1,1
  2. (−1,1)
  3. [−3,−2]∪[2,3]
  4. [−5,5]
5.2.6.21.
Answer.
  1. −2, 2
  2. −2.8, 0, 2.8
  3. −2.5<q<−1.25 and 1.25<q<2.5
  4. −2<q<0 and 2<q
5.2.6.23.
Answer.
  1. g(−6)=0, g(6)=0, g(0)=6
  2. none
  3. g(x) is undefined for those x-values
5.2.6.25.
Answer.
  1. 0, 5
  2. 0, −32
  3. 56
  4. −5, 12
  5. parabola and line

5.3 Some Basic Graphs
5.3.4 Problem Set 5.3

Warm Up

5.3.4.1.
Answer.
  1. 4
  2. 2
5.3.4.3.
Answer.
  1. 2.080
  2. 6.366
  3. −0.126
  4. −1.458
5.3.4.5.
Answer.
  1. 12
  2. −13
5.3.4.7.
Answer.
  1. −9
  2. 9
  3. 9
  4. −4
5.3.4.9.
Answer.
  1. −50
  2. −43
  3. 144

Skills Practice

5.3.4.11.
Answer.
grid
5.3.4.13.
Answer.
square root of x
5.3.4.15.
Answer.
grid
5.3.4.17.
Answer.
  1. f
  2. g

Applications

5.3.4.19.
Answer.
(−∞,0)∪[0.5,∞)
5.3.4.21.
Answer.
(b): 2 units down, (c): 1 unit up
5.3.4.23.
Answer.
(b): 1.5 units left, (c): 1 unit right
5.3.4.25.
Answer.
(b): reflected about x-axis, (c): reflected about y-axis
5.3.4.27.
Answer.
  1. vi
  2. ii
  3. iv
  4. i
  5. v
  6. iii
5.3.4.29.
Answer.
  1. horizontal shift of square root y=x
  2. vertical shift of cube root y=x3
  3. vertical shift of absolute value y=|x|
  4. vertical flip of reciprocal y=1x
  5. vertical flip and vertical shift of cube y=x3
  6. vertical flip and vertical shift of inverse-square y=1x2
5.3.4.31.
Answer.
  1. x<2
  2. x>−23
5.3.4.33.
Answer.
  1. 41
  2. no solution
  3. 29<x≤61

5.4 Direct Variation
5.4.6 Problem Set 5.4

Warm Up

5.4.6.1.
Answer.
  1. −10
  2. −16,20

Skills Practice

5.4.6.3.
Answer.
  1. y=0.3x
  2. x 2 5 8 12 15
    y −0.6 1.5 2.4 3.6 4.5
  3. y doubles also
5.4.6.5.
Answer.
(b), k=0.5
5.4.6.7.
Answer.
(c)

Applications

5.4.6.9.
Answer.
  1. Price of item 18 28 12
    Tax 1.17 1.82 0.78
    Tax/Price 0.065 0.065 0.065
    Yes; 6.5%
  2. T=0.065p
  3. direct variation
5.4.6.11.
Answer.
  1. m=0.165w
    w 50 100 200 400
    m 8.25 16.5 33 66
  2. 19.8 lb
  3. 303.03 lb
  4. It will double.
5.4.6.13.
Answer.
  1. v=15.306d
  2. 3985 million light-years
  3. 18,979 km/sec
5.4.6.15.
Answer.
  1. P=18258192w3≈0.228w3
    w 10 20 40 80
    P 223 1782 14,259 114,074
  2. 752 kilowatts
  3. 33.54 mph
  4. It is multiplied by 8.
5.4.6.17.
Answer.
  1. d=0.005v2
  2. 50 m
5.4.6.19.
Answer.
  1. W=600d2
  2. 864 newtons
5.4.6.20.
Answer.
  1. Wind resistance quadruples.
  2. It is one-ninth as great.
  3. It is decreased by 19% because it is 81% of the original.

5.5 Inverse Variation
5.5.4 Problem Set 5.5

Warm Up

5.5.4.1.
Answer.
R=13I. Not inverse variation.
5.5.4.3.
Answer.
W=32,000−d Not inverse variation.

Skills Practice

5.5.4.5.
Answer.
(c)
5.5.4.7.
Answer.
  1. y=120x
  2. x 4 8 20 30 40
    y 30 15 6 4 3
  3. y is divided by 2
5.5.4.9.
Answer.
(b), k=72
5.5.4.11.
Answer.
(c)

Applications

5.5.4.13.
Answer.
  1. Width (feet) 2 2.5 3
    Length (feet) 12 9.6 8
    Length×width 24 24 24
    24 square feet
  2. L=24w
  3. inverse variation
5.5.4.15.
Answer.
  1. B=88d
    d 1 2 12 24
    B 88 44 7.3 3.7
  2. 8.8 milligauss
  3. More than 20.47 in
  4. It is one half as strong.
5.5.4.17.
Answer.
  1. 645
  2. D=64,500n
  3. 215
5.5.4.18.
Answer.
  1. m=8p
  2. 0.8 ton
5.5.4.20.
Answer.
  1. T=4000d
  2. 8∘C
5.5.4.22.
Answer.
  1. It is one-fourth the original illumination.
  2. It is one-ninth the illumination.
  3. It is 64% of the illumination.

5.6 Functions as Models
5.6.6 Problem Set 5.6

Warm Up

5.6.6.1.
Answer.
  1. (0,∞)
  2. none
  3. (−infty,0)
  4. none
5.6.6.3.
Answer.
  1. none
  2. (0,∞)
  3. none
  4. none
5.6.6.5.
Answer.
  1. (−infty,0)
  2. none
  3. (0,∞)
  4. none
5.6.6.7.
Answer.
  1. s=h(t)
  2. After 3 seconds, the duck is at a height of 7 meters.

Skills Practice

5.6.6.9.
Answer.
  1. Increasing
  2. Concave up
5.6.6.11.
Answer.
y=kx

Applications

5.6.6.13.
Answer.
(b)
5.6.6.15.
Answer.
(a)
5.6.6.17.
Answer.
(b)
5.6.6.19.
Answer.
  1. II
  2. IV
  3. I
  4. III
5.6.6.21.
Answer.
y=x3 stretched or compressed vertically
cubic
5.6.6.23.
Answer.
y=1x stretched or compressed vertically
reciprocal
5.6.6.25.
Answer.
y=x
root
5.6.6.27.
Answer.
  1. Table (4), Graph (C)
  2. Table (3), Graph (B)
  3. Table (1), Graph (D)
  4. Table (2), Graph (A)
5.6.6.29.
Answer.
  1. III
  2. 3

Absolute Value

5.6.6.1.
Answer.
  1. |x|=6
  2. number line
5.6.6.3.
Answer.
  1. |p+3|=5
  2. number line
5.6.6.5.
Answer.
  1. |t−6|<3
  2. number line
5.6.6.7.
Answer.
  1. |b+1|≥0.5
  2. number line
5.6.6.9.
Answer.
absolute value graph
  1. x=−5 or x=−1
  2. −7≤x≤1
  3. x<−8 or x>2
5.6.6.11.
Answer.
absolute value graph
  1. x=4
  2. No solution
  3. No solution
5.6.6.13.
Answer.
x=−32 or x=52
5.6.6.15.
Answer.
q=−73
5.6.6.17.
Answer.
b=−14 or b=10
5.6.6.19.
Answer.
w=132 or w=152
5.6.6.21.
Answer.
No solution
5.6.6.23.
Answer.
No solution
5.6.6.25.
Answer.
−92<x<−32
5.6.6.27.
Answer.
d≤−2  or  d≥5
5.6.6.29.
Answer.
All real numbers
5.6.6.31.
Answer.
1.4<t<1.6
5.6.6.33.
Answer.
T≤3.2  or  T≥3.3
5.6.6.35.
Answer.
No solution

5.7 Chapter 5 Summary and Review
5.7.3 Chapter 5 Review Problems

5.7.3.1.

Answer.
A function: Each x has exactly one associated y-value.

5.7.3.2.

Answer.
Not a function

5.7.3.3.

Answer.
Not a function: The IQ of 98 has two possible SAT scores.

5.7.3.4.

Answer.
A function

5.7.3.5.

Answer.
N(10)=7000: Ten days after the new well is opened, the company has pumped a total of 7000 barrels of oil.

5.7.3.6.

Answer.
H(16)=3: At 16 mph, the trip takes 3 hours.

5.7.3.7.

Answer.
F(0)=1,  F(−3)=37

5.7.3.8.

Answer.
G(0)=−2,  G(20)=123

5.7.3.9.

Answer.
h(8)=−6,  h(−8)=−14

5.7.3.10.

Answer.
m(5)=6,  m(−40)=−4.8

5.7.3.11.

Answer.
  1. P(0)=5
  2. x=5,~x=1

5.7.3.12.

Answer.
  1. R(0)=2
  2. x=2, x=−2

5.7.3.13.

Answer.
  1. f(−2)=3,  f(2)=5
  2. t=1,  t=3
  3. t-intercepts (−3,0),(4,0); f(t)-intercept: (0,2)
  4. Maximum value of 5 occurs at t=2

5.7.3.14.

Answer.
  1. P(−3)=−2,  P(3)=3
  2. z=−5, −12, 4
  3. (−4,0),(−1,0),(5,0); (0,3)
  4. Maximum value of −3 occurs at z=−2

5.7.3.15.

Answer.
Function

5.7.3.16.

Answer.
not a function

5.7.3.17.

Answer.
Not a function

5.7.3.18.

Answer.
Function

5.7.3.19.

Answer.
line

5.7.3.21.

Answer.
line

5.7.3.23.

Answer.
  1. x=12=0.5
  2. x=278≈3.4
  3. x>4.9
  4. x≤2.0

5.7.3.24.

Answer.
  1. x=0.4
  2. x=3.2
  3. 0<x≤4.5
  4. x<0 or x>0.2

5.7.3.25.

Answer.
  1. x≈±5.8
  2. x=±0.4
  3. −2.5<x<0 or 0<x<2.5
  4. x≤−0.5 or x≥0.5

5.7.3.26.

Answer.
  1. x=0.5
  2. x=2.9
  3. 0≤x<2.3
  4. x≥1.7

5.7.3.27.

Answer.
y=1.2x2

5.7.3.28.

Answer.
y=54x

5.7.3.29.

Answer.
y=20x

5.7.3.30.

Answer.
y=720x2

5.7.3.31.

Answer.
  1. d=1.75t2
  2. 63 cm

5.7.3.32.

Answer.
  1. V=4TP
  2. 32

5.7.3.33.

Answer.
480 bottles

5.7.3.34.

Answer.
14.0625 lumens

5.7.3.35.

Answer.
  1. w=kr2
  2. inverse square in first quadrant
  3. 39603≈6860 miles

5.7.3.37.

Answer.
curve

5.7.3.38.

Answer.
oven temprature

5.7.3.39.

Answer.
inverse square

5.7.3.41.

Answer.
inverse square

5.7.3.43.

Answer.
  1. curve
  2. g(x)=24x

5.7.3.44.

Answer.
  1. decreasing cubic
  2. F(x)=−x3

5.7.3.45.

Answer.
  1. x 0 4 8 14 16 22
    y 24 20 16 10 8 2
  2. y=24−x

5.7.3.46.

Answer.
  1. x 0 4 10 12 14 16
    y 0 6 15 18 21 24
  2. y=32x

5.7.3.47.

Answer.
  1. x 0 1 4 9 16 25
    y 0 1 2 3 4 5
  2. y=x

5.7.3.48.

Answer.
  1. x 0.25 0.50 1.00 1.50 2.00 4.00
    y 4.00 2.00 1.00 0.67 0.50 0.25
  2. y=1x

5.7.3.49.

Answer.
  1. x −3 −2 −1 0 1 2
    y 5 0 −3 −4 −3 0
  2. y=x2−4

5.7.3.50.

Answer.
  1. x −3 −2 −1 0 1 4
    y 0 5 8 9 8 −7
  2. y=9−x2

6 Powers and Roots
6.1 Integer Exponents
6.1.5 Problem Set 6.1

Warm Up

6.1.5.1.
Answer.
  1. −2z2
  2. −24z4
6.1.5.3.
Answer.
  1. −12x3y4
  2. 14ab4
6.1.5.5.
Answer.
  1. 4x10y14
  2. x4y

Skills Practice

6.1.5.7.
Answer.
  1. 9
  2. 19
  3. 9
  4. 19
  5. −9
  6. −19
6.1.5.9.
Answer.
  1. 40
  2. 58
  3. 58
  4. 140
  5. 40
  6. 85
6.1.5.11.
Answer.
  1. 34
  2. 18
  3. 12
  4. 94
  5. 16
  6. 2
  7. 43
  8. 8
6.1.5.13.
Answer.
  1. 1(m−n)2
  2. 1y2+1y3
  3. 2pq4
  4. −5x5y2
6.1.5.15.
Answer.
1.25
6.1.5.17.
Answer.
0.2
6.1.5.19.
Answer.
  1. 20x3
  2. 13u12
  3. 58t
6.1.5.21.
Answer.
  1. 13x+3x−1
  2. 14x−2−32x−1
6.1.5.23.
Answer.
x−3+2x−1
6.1.5.25.
Answer.
−4−2u−1+6u−2
6.1.5.27.
Answer.
4x−2(x4+4)
6.1.5.29.
Answer.
  1. 2.85×102
  2. 8.372×106
  3. 2.4×10−2
  4. 5.23×10−4

Applications

6.1.5.31.
Answer.
  1. x 1 2 4.5 6.2 9.3
    g(x) 1 0.125 0.011 0.0042 0.0012
  2. they decrease
  3. x 1.5 0.6 0.1 0.03 0.002
    f(x) 0.30 4.63 1000 37,037 125×106
  4. they increase
6.1.5.33.
Answer.
  1. P=0.355v3
  2. v≈52.03 mph
  3. 3.375
6.1.5.35.
Answer.
  1. d=50f−1
  2. The are of the aperture decreases by a factor of 0.5 at each f-stop.
6.1.5.36.
Answer.
  1. 1.905×1013
  2. $57,552.87

6.2 Roots and Radicals
6.2.9 Problem Set 6.2

Warm Up

6.2.9.1.
Answer.
13; 4; 3; 10; 6; 7
6.2.9.3.
Answer.
  1. 1.414
  2. 4.217
  3. 1.125
  4. 0.140
  5. 2.782
  6. 3.162

Skills Practice

6.2.9.5.
Answer.
  1. −3
  2. undefined
  3. −3
  4. −3
6.2.9.7.
Answer.
  1. 7
  2. 3x4
  3. 3x4
6.2.9.9.
Answer.
  1. 51/2
  2. (4y)1/3
  3. 5x1/3
6.2.9.11.
Answer.
14x1/2−2x−1/2+12x
6.2.9.13.
Answer.
x0.5+x−0.25−1
6.2.9.15.
Answer.
x=91.125
6.2.9.17.
Answer.
x=192
6.2.9.19.
Answer.
x=±30
6.2.9.21.
Answer.
L=gT24π2
6.2.9.23.
Answer.
M=d3m16r2
6.2.9.25.
Answer.
A=EST4
6.2.9.27.
Answer.
  1. I
  2. III
  3. II
  4. III
6.2.9.29.
Answer.
  1. G(x)=3.7x1/3
  2. H(x)=851/4x1/4
  3. F(t)=25t−1/5

Applications

6.2.9.31.
Answer.
  1. L (feet) 200 400 600 800 1000
    vmax (knots) 18.4 26 31.8 36.8 41.1
  2. maximum velocity
  3. 50.4 knots
  4. maximum and cruise velocities
  5. 31 knots, 62%
6.2.9.33.
Answer.
  1. T=2π32L1/2
  2. 10.54 sec
  3. square root
6.2.9.35.
Answer.
  1. $87.68; $72.00
  2. 1989; 2013
6.2.9.36.
Answer.
  1. 6.5×10−13 cm; 1.17×10−36 cm3
  2. 1.8×1014g/cm3
  3. Element Carbon Potassium Cobalt Technetium Radium
    Mass
    number, A     		14 40 60 99 226
    Radius, r
    (10−13 cm)    		 3.1 4.4 5.1 6 7.9
  4. cube root
6.2.9.37.
Answer.
132.6 km
6.2.9.39.
Answer.
  1. cube root
  2. x=36

6.3 Rational Exponents
6.3.7 Problem Set 6.3

Warm Up

6.3.7.1.
Answer.
  1. 4; 4
  2. 15.59; 15.59
  3. 8; −81
6.3.7.3.
Answer.
  1. 2y3
  2. 3x2
  3. 2x2y9
  4. −3a2b3
  5. 4x2y6
  6. −2x5y

Skills Practice

6.3.7.5.
Answer.
  1. y34
  2. 1a27
  3. 1s3t35
6.3.7.7.
Answer.
  1. y3/2
  2. 6a3/5b3/5
  3. −2nq−11/8
6.3.7.9.
Answer.
4a2
6.3.7.11.
Answer.
4w3/2
6.3.7.13.
Answer.
12k1/4
6.3.7.15.
Answer.
x=64
6.3.7.17.
Answer.
x=1.157
6.3.7.19.
Answer.
29.524
6.3.7.21.
Answer.
133
6.3.7.23.
Answer.
2x−x2/3
6.3.7.25.
Answer.
2x1/2−x1/4−1
6.3.7.27.
Answer.
x(x1/2+1)
6.3.7.29.
Answer.
a2/3+a1/3−1a1/3
6.3.7.31.
Answer.
x 0 1 2 3 4 5 6
f(x) 0 1 2.5 4.3 6.4 8.5 10.9
g(x) 0 1 2.8 5.2 8 11.2 14.7
power functions
6.3.7.33.
Answer.
  1. 2.83
  2. 3.30

Applications

6.3.7.35.
Answer.
  1. t 5 10 15 20
    I(t) 131 199 254 302
  2. 20 days
  3. graph
6.3.7.37.
Answer.
  1. A 10 100 1000 5000 10,000
    S 25 42 69 98 115
  2. power function
  3. 81, 71
  4. 126,000 sq km
6.3.7.39.
Answer.
  1. 1.62
  2. 1.62
  3. 0.84; 0.84
6.3.7.41.
Answer.
  1. 15 days, 28 days
  2. I(m)×W(m)m=0.18m−0.041
  3. m−0.041 is close to 1
6.3.7.43.
Answer.
  1. p=K1/2a3/2
  2. 1.88 years

6.4 Working with Radicals
6.4.6 Problem Set 6.4

Warm Up

6.4.6.1.
Answer.
No
6.4.6.3.
Answer.
Yes
6.4.6.5.
Answer.
 ab=ab 
 ab=ab 

Skills Practice

6.4.6.7.
Answer.
  1. 32
  2. 233
  3. −244=−22
6.4.6.9.
Answer.
  1. x3x3
  2. 3z3z
  3. 2a23a4
6.4.6.11.
Answer.
  1. 24−x2
  2. A8+A33
6.4.6.13.
Answer.
  1. ab23
  2. xxy
6.4.6.15.
Answer.
  1. 433
  2. −23
6.4.6.17.
Answer.
  1. 63+65
  2. 3k3−3k22
6.4.6.19.
Answer.
  1. x−9
  2. −4+6
6.4.6.21.
Answer.
  1. 3−5
  2. −4+22
  3. 2a−22a
6.4.6.23.
Answer.
  1. 14x6
  2. 2abb
6.4.6.25.
Answer.
  1. −21−3
  2. x(x+3x2−3

Applications

6.4.6.27.
Answer.
x2+4x=(4−45+5)+(8+45)=1
6.4.6.29.
Answer.
  1. w32
  2. w234

6.5 Radical Equations
6.5.6 Problem Set 6.5

Warm Up

6.5.6.1.
Answer.
  1. z=4
  2. w=8
6.5.6.3.
Answer.
  1. square root graph
  2. 13
6.5.6.5.
Answer.
  1. x y
    −25 6.92
    −20 6.71
    −15 6.47
    −10 6.15
    −5 3.21
    0 4
    5 2.29
    10 1.85
    15 1.53
    20 1.29
    grid
  2. −8

Skills Practice

6.5.6.7.
Answer.
−13
6.5.6.9.
Answer.
5
6.5.6.11.
Answer.
5
6.5.6.13.
Answer.
16
6.5.6.15.
Answer.
31
6.5.6.17.
Answer.
  1. 2|x|
  2. |x−5|
  3. |x−3|

Applications

6.5.6.19.
Answer.
  1. 1.25 mi; 6600 ft
  2. h=(d89.4)2
6.5.6.21.
Answer.
  1. 339.39 cubic in
  2. V=12.57r3
6.5.6.23.
Answer.
b=±a2−c2
6.5.6.25.
Answer.
W=v1−(DS)3

6.6 Chapter 6 Summary and Review
6.6.3 Chapter 6 Review Problems

6.6.3.1.

Answer.
  1. 181
  2. 164

6.6.3.2.

Answer.
  1. 9
  2. 75

6.6.3.3.

Answer.
  1. 1243m5
  2. −7y8

6.6.3.4.

Answer.
  1. 1a+1a2
  2. 3r2q9

6.6.3.5.

Answer.
  1. 2c3
  2. 99z2

6.6.3.6.

Answer.
  1. d816k12
  2. 25w14

6.6.3.7.

Answer.
  1. 1.018×10−9 sec, or 0.000 000 001 018 sec
  2. 8 min 20 sec

6.6.3.8.

Answer.
1,200,000,000,000 hr, or 78,904,109,590 yr

6.6.3.9.

Answer.
  1. 5000 sec, or 83 min
  2. 110
  3. 42 yr

6.6.3.10.

Answer.
  1. Planet Density
    Mercury 5426
    Venus 5244
    Earth 5497
    Mars 3909
    Jupiter 1241
    Saturn 620
    Uranus 1238
    Neptune 1615
    Pluto 2355
  2. Mercury, Venus, Earth, and Mars

6.6.3.11.

Answer.
  1. 25m
  2. 8n3

6.6.3.12.

Answer.
  1. (13d)33
  2. 6x2y35

6.6.3.13.

Answer.
  1. 127q34
  2. 7u3v3

6.6.3.14.

Answer.
  1. a2+b2
  2. 16−x24

6.6.3.15.

Answer.
  1. 2x2/3
  2. 14x1/4

6.6.3.16.

Answer.
  1. z5/2
  2. z4/3

6.6.3.17.

Answer.
  1. 6b−3/4
  2. −13b−1/3

6.6.3.18.

Answer.
  1. −4a−1/2
  2. 2a−3/2

6.6.3.19.

Answer.
112 kg

6.6.3.20.

Answer.
Height: 2.673 in; diameter: 5.346 in

6.6.3.21.

Answer.
  1. 480
  2. 498

6.6.3.22.

Answer.
  1. 294 sq in
  2. 90 lb

6.6.3.23.

Answer.
  1. increasing concave down
  2. 283
  3. 2051

6.6.3.25.

Answer.
  1. power function
  2. $7114.32

6.6.3.27.

Answer.
  1. It is the cost of producing the first ship.
  2. C=12x8 million
  3. About $11 million; about 8.3% ; about 8.3%>
  4. About 8.3%

6.6.3.29.

Answer.
  1. 4096
  2. 18
  3. 363≈62.35
  4. 400,000

6.6.3.30.

Answer.
  1. −27
  2. −34
  3. −34003≈−22.1
  4. −300

6.6.3.31.

Answer.
169

6.6.3.32.

Answer.
49

6.6.3.33.

Answer.
16

6.6.3.34.

Answer.
−16

6.6.3.35.

Answer.
1, 4

6.6.3.36.

Answer.
8

6.6.3.37.

Answer.
9

6.6.3.38.

Answer.
12

6.6.3.39.

Answer.
7

6.6.3.40.

Answer.
±8

6.6.3.41.

Answer.
5

6.6.3.42.

Answer.
4

6.6.3.43.

Answer.
g=2vt2

6.6.3.44.

Answer.
r=±3q2−6q+72

6.6.3.45.

Answer.
p=±2R2−R

6.6.3.46.

Answer.
r=±2q3−1

6.6.3.47.

Answer.
  1. 5p4a25p
  2. 2w23v23

6.6.3.48.

Answer.
  1. a2b
  2. xy

6.6.3.49.

Answer.
  1. 2a3−2b63
  2. −4ab223

6.6.3.50.

Answer.
  1. 2t1+6t4
  2. 4t46

6.6.3.51.

Answer.
  1. x2−4xx+4x
  2. x2−4x

6.6.3.52.

Answer.
  1. 14−46
  2. 2a−4b

6.6.3.53.

Answer.
  1. 75y5y
  2. 32d

6.6.3.54.

Answer.
  1. 33rs11s
  2. 13mm

6.6.3.55.

Answer.
  1. −3a+6a−4
  2. −3z−12z−16

6.6.3.56.

Answer.
  1. 2x2+x3−3x2−3
  2. 5m2−7m3+625m2−12

7 Exponential Functions
7.1 Exponential Growth and Decay
7.1.7 Problem Set 7.1

Warm Up

7.1.7.1.
Answer.
  1. $28
  2. #31.36
  3. No. It increase by 12% of different amounts.
7.1.7.3.
Answer.
Decreased by 1%.
7.1.7.5.
Answer.
4
7.1.7.7.
Answer.
±1.2
7.1.7.9.
Answer.
−2.14; 0.14

Skills Practice

7.1.7.11.
Answer.
  1. P=1200+150t; 1650
  2. P=1200⋅1.5t; 4050
7.1.7.13.
Answer.
  1. V=18,000−2000t; $8000
  2. V=18,000⋅0.8t; $5898.24
7.1.7.15.
Answer.
20%, 2%, 7.5%, 100%, 115%
7.1.7.17.
Answer.
  1. P0=4, b=21/3
  2. P(t)=4⋅2t/3
7.1.7.18.
Answer.
  1. Initial value 80, decay factor 12
  2. f(x)=80⋅(12)x
7.1.7.19.
Answer.
The growth factor is 1.2.
x 0 1 2 3 4
Q 20 24 28.8 34.56 41.47
7.1.7.20.
Answer.
The decay factor is 0.8.
w 0 1 2 3 4
N 120 96 76.8 61.44 49.15
7.1.7.21.
Answer.
The decay factor is 0.8.
t 0 1 2 3 4
C 10 8 6.4 5.12 4.10
7.1.7.22.
Answer.
The growth factor is 1.1.
n 0 1 2 3 4
B 200 220 242 266.2 292.82

Applications

7.1.7.23.
Answer.
  1. Years after 2010 0 1 2 3 4
    Windsurfers 1500 1680 1882 2107 2360
  2. S(t)=1200(1.12)t
  3. Windsurfer sales exponential growth
  4. 2644; 5844
7.1.7.24.
Answer.
  1. Years after 1983 0 5 10 15 20
    Value of house 20,000 25,526 32,578 41,579 53,066
  2. V(t)=200,000(1.05)t
  3. house value
  4. $359,171.27; $458,403.66
7.1.7.25.
Answer.
  1. Weeks 0 6 12 18 24
    Bees 2000 5000 12,500 31,250 78,125
  2. P(t)=2000(2.5)t/6
  3. bee population
7.1.7.27.
Answer.
  1. Weeks 0 2 4 6 8
    Mosquitos 250,000 187,500 140,625 105,469 79,102
  2. P(t)=250,000(0.75)t/2
  3. graph
  4. 162,280; 68,504
7.1.7.29.
Answer.
  1. Years 0 3 6 9 12
    Value of boat 15,000 13,500 12,150 10,935 9841.50
  2. V(t)=15,000(0.885)t
  3. motorboat depreciation
  4. $4995.52; $4421.04
7.1.7.31.
Answer.
  1. P(t)=1,545,387bt
  2. b=1.049; r=4.9%
  3. 3,167,157
7.1.7.33.
Answer.
  1. 365
  2. exponential decay
  3. N(t)=365(0.356)t
  4. 0.03
7.1.7.35.
Answer.
  1. 2440 tigers per decade
  2. 0.765; 23.5%
  3. 3080; 4656
7.1.7.37.
Answer.
  1. 39; 1.045
  2. 36; 1.047
  3. Species B

7.2 Exponential Functions
7.2.6 Problem Set 7.2

Warm Up

7.2.6.1.
Answer.
  1. 3x+4
  2. 34x
  3. 12x
7.2.6.3.
Answer.
  1. b−2t
  2. bt/2
  3. 1
7.2.6.5.
Answer.
0.06

Skills Practice

7.2.6.7.
Answer.
23
7.2.6.8.
Answer.
−14
7.2.6.9.
Answer.
17
7.2.6.11.
Answer.
−54
7.2.6.13.
Answer.
±2
7.2.6.15.
Answer.
2.26
7.2.6.17.
Answer.
  1. (0,26); increasing
  2. (0,1.2); decreasing
  3. (0,75); decreasing
  4. (0,23); increasing
7.2.6.18.
Answer.
  1. Power
  2. Exponential
  3. Power
  4. Neither
7.2.6.19.
Answer.
x −3 −2 −1 0 1 2 3
f(x)=3x 127 19 13 1 3 9 27
g(x)=(13)x 27 9 3 1 13 19 127
two exponentials
7.2.6.21.
Answer.
Because they are defined by equivalent expressions, (b), (c), and (d) have identical graphs.
7.2.6.23.
Answer.
0.17;5.95
7.2.6.25.
Answer.
  1. P0=800
  2. x 0 1 2
    g(x) 800 200 50
  3. a=14
  4. g(x)=800(14)x
7.2.6.27.
Answer.
  1. Power y=100x−1
  2. Exponential P=14⋅2x

Applications

7.2.6.28.
Answer.
  1. V(t)=20,000(0.8)t/3
  2. GC decay
  3. 6 years
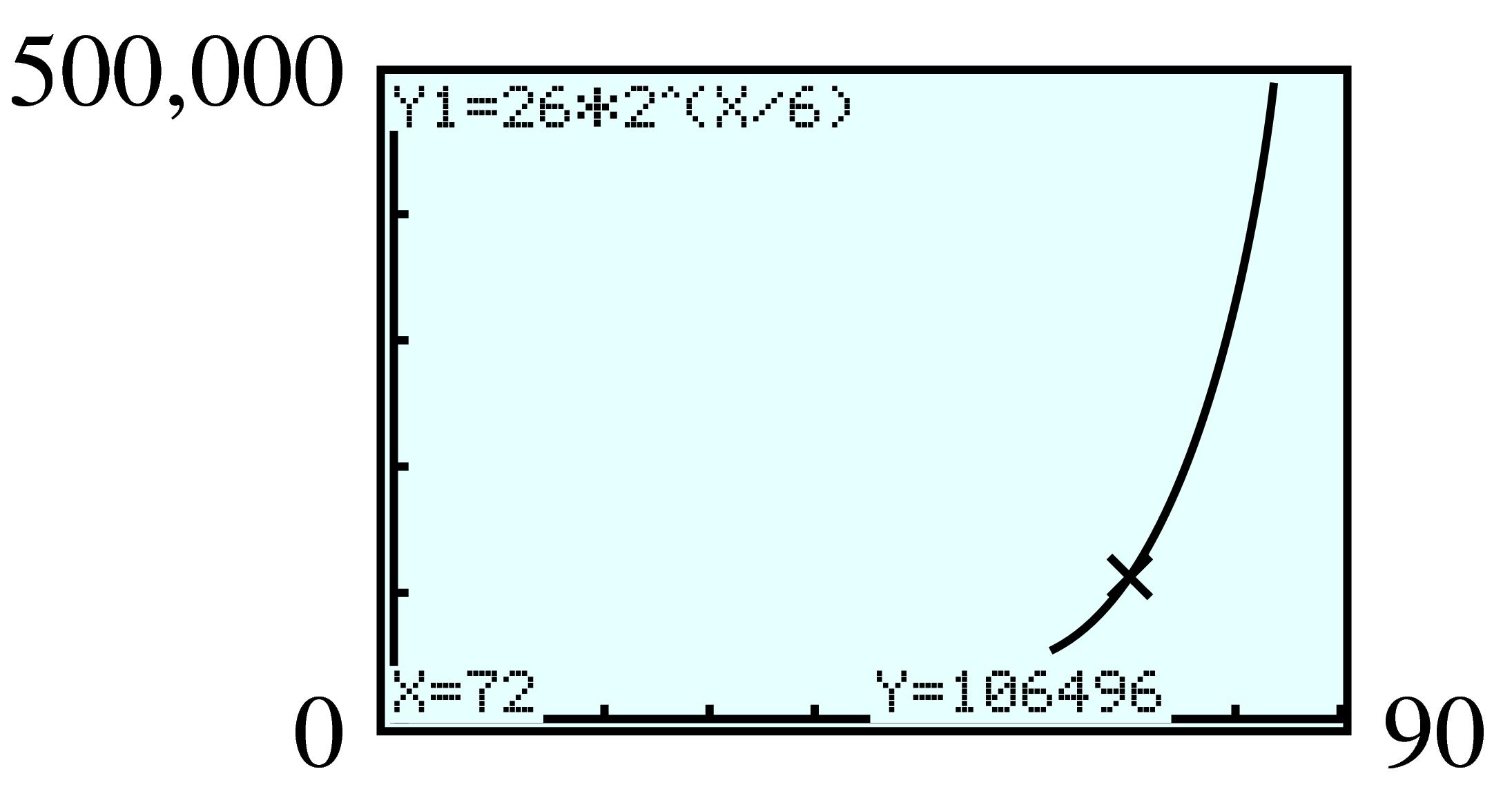
7.2.6.29.
Answer.
  1. N(t)=26(2)t/6
  2. GC growth
  3. 72 days later
7.2.6.30.
Answer.
  1. F0=400
  2. b=1.06
  3. F(p)=440(1.06)p
7.2.6.31.
Answer.
  1. S0=150
  2. b≈0.55
  3. S(d)=150(0.55)d
7.2.6.32.
Answer.
x f(x)=x2 g(x)=2x
−2 4 14
−1 1 12
0 0 1
1 1 2
2 4 4
3 9 8
4 16 16
5 25 32
exponential growth and qudratic
  1. Three
  2. x=−0.77, 2, 4
  3. (−0.77,2)∪(4,∞)
  4. g(x)

7.3 Logarithms
7.3.7 Problem Set 7.3

Warm Up

7.3.7.1.
Answer.
  1. P(t)=300(1.15)t
  2. 500=300(1.15)t
  3. t≈3.65

Skills Practice

7.3.7.3.
Answer.
  1. 2
  2. 4
7.3.7.5.
Answer.
  1. 12
  2. −1
7.3.7.7.
Answer.
  1. −1
  2. 4
7.3.7.9.
Answer.
  1. 3<x<4
  2. −1<y<0
7.3.7.11.
Answer.
−0.23
7.3.7.13.
Answer.
0.77
7.3.7.15.
Answer.
2.53
7.3.7.17.
Answer.
  1. logt⁡(16)=32
  2. log0.8⁡(M)=1.2
7.3.7.19.
Answer.
  1. 16w=256
  2. b^{-2} = 9
7.3.7.21.
Answer.
  1. x=log4⁡(2.5)≈2.7
  2. x=log2⁡(0.2)≈−2.3
7.3.7.23.
Answer.
2.77

Applications

7.3.7.25.
Answer.
  1. 2030
  2. 6.2%
7.3.7.27.
Answer.
  1. 9.60 in
  2. 3.85 mi
7.3.7.29.
Answer.
  1. 2,018,436
  2. 5.17%
  3. 2008
7.3.7.31.
Answer.
  1. 4
  2. 4
  3. 64
  4. 163≈2.52

7.4 Properties of Logarithms
7.4.5 Problem Set 7.4

Warm Up

7.4.5.1.
Answer.
  1. log8⁡(12)=−13
  2. log5⁡(46)=x
7.4.5.3.
Answer.
  1. 108
  2. 2; 6; 8  Property (1)
7.4.5.5.
Answer.
  1. b3
  2. 8; 5; 3  Property (2)
7.4.5.7.
Answer.
  1. 1015
  2. 15; 3  Property (3)
7.4.5.9.
Answer.
  1. 5
  2. 6
  3. 5
(a) and (c) are equal.
7.4.5.11.
Answer.
  1. log⁡(24)≈1.38
  2. log⁡(240)≈2.38
  3. log⁡(230)≈2.36
None are equal.

Skills Practice

7.4.5.13.
Answer.
  1. logb⁡(2)+logb⁡(x)
  2. logb⁡(2)−logb⁡(x)
  3. 3logb⁡(x)
  4. 23logb⁡(x)
7.4.5.15.
Answer.
  1. log3⁡(3)+4log3⁡(x)
  2. (1/t)log5⁡(1.1)
  3. Extra \left or missing \right
  4. log2⁡(5)+x
7.4.5.17.
Answer.
  1. logb⁡(4)
  2. log4⁡(x2y3)
7.4.5.19.
Answer.
  1. log⁡(2x5/2)
  2. log⁡(t−4)
7.4.5.21.
Answer.
y=125
7.4.5.23.
Answer.
b=100
7.4.5.25.
Answer.
2.81
7.4.5.27.
Answer.
−1.61
7.4.5.29.
Answer.
−12.49
7.4.5.31.
Answer.
  1. 1.7918
  2. −0.9163
7.4.5.33.
Answer.
(a) and (d)
7.4.5.35.
Answer.
(a) and (c)

Applications

7.4.5.37.
Answer.
  1. S(t)=S0(1.09)t
  2. 4.7 hours
7.4.5.39.
Answer.
  1. C(t)=0.7(0.80)t
  2. After 2.5 hours
  3. decay
7.4.5.41.
Answer.
  1. S(t)=S0⋅0.9527t
  2. 28.61 hours
7.4.5.43.
Answer.
k=log⁡(NN0)tlog⁡(a)
7.4.5.45.
Answer.
t=1klog⁡(AA0+1)

7.5 Exponential Models
7.5.5 Problem Set 7.5

Warm Up

7.5.5.1.
Answer.
4.16
7.5.5.3.
Answer.
16
7.5.5.5.
Answer.
y=−23x−1
7.5.5.7.
Answer.
y=−12x+4

Skills Practice

7.5.5.9.
Answer.
P(x)=8(0.5)x
7.5.5.11.
Answer.
y=1.5(3)x/5
7.5.5.13.
Answer.
  1. y=2.6−1.3x
  2. y=2.5(0,5)x
  3. graph

Applications

7.5.5.15.
Answer.
P(t)=2000(2t/5;  14.9%
7.5.5.17.
Answer.
D(t)=D0(0,5t/18;  3.8%
7.5.5.19.
Answer.
  1. P=P0(2)t/25
  2. 2.81%
7.5.5.21.
Answer.
  1. log⁡(0.5)log⁡(0.946)≈12.5 hours
  2. 25 hours
  3. decay
7.5.5.23.
Answer.
  1. D(t)=D0(0.5)t/15
  2. After 89.5 years, or in 2060
7.5.5.25.
Answer.
  1. A=A0(12)t/5730
  2. About 760 years old
7.5.5.27.
Answer.
12.9%
7.5.5.29.
Answer.
About 11 years

7.6 Chapter 7 Summary and Review
7.6.3 Chapter 7 Review Problems

7.6.3.1.

Answer.
  1. D=8(1.5)t/5
  2. 18; 44

7.6.3.3.

Answer.
  1. M=100(0.85)t
  2. 52.2 mg; 19.7 mg

7.6.3.5.

Answer.
growth

7.6.3.7.

Answer.
growth

7.6.3.9.

Answer.
−43

7.6.3.11.

Answer.
−11

7.6.3.13.

Answer.
4

7.6.3.15.

Answer.
−1

7.6.3.17.

Answer.
−3

7.6.3.19.

Answer.
2x−2=3

7.6.3.20.

Answer.
np−1=q

7.6.3.21.

Answer.
log0.3⁡(x+1)=−2

7.6.3.23.

Answer.
−1

7.6.3.25.

Answer.
4

7.6.3.27.

Answer.
log⁡(5.1)1.3≈0.5433

7.6.3.29.

Answer.
log⁡(2.9/3)−0.7≈0.21

7.6.3.31.

Answer.
0.054

7.6.3.32.

Answer.
2.959

7.6.3.33.

Answer.
0.195

7.6.3.34.

Answer.
2.823

7.6.3.35.

Answer.
logb⁡(x)+13logb⁡(y)−2logb⁡(z)

7.6.3.37.

Answer.
43log⁡(x)−13log⁡(y)

7.6.3.39.

Answer.
Missing \left or extra \right

7.6.3.41.

Answer.
log⁡(18)

7.6.3.43.

Answer.
log⁡(63)log⁡(3)≈3.77

7.6.3.45.

Answer.
log⁡(50)−0.3log⁡(6)≈−7.278

7.6.3.47.

Answer.
log⁡(N/N0)k

7.6.3.49.

Answer.
  1. 238
  2. 2010

7.6.3.51.

Answer.
  1. C=90(1.06)t
  2. $94.48
  3. 5 years

8 Polynomial and Rational Functions
8.1 Polynomial Functions
8.1.10 Problem Set 8.1

Warm Up

8.1.10.1.
Answer.
  1. a2+2ab+b2
  2. a2−2ab+b2
8.1.10.3.
Answer.
  1. (x−7)2
  2. cannot be factored
  3. (x+3)2
  4. (x+8)(x−8)

Skills Practice

8.1.10.5.
Answer.
(b) and (c) are not polynomials; they have variables in a denominator.
8.1.10.7.
Answer.
  1. −1.9x3+x+6.4
  2. −2x2+6xy+2y3
8.1.10.9.
Answer.
  1. 4
  2. 5
  3. 7
8.1.10.11.
Answer.
  1. 6a4−5a3−5a2+5a−1
  2. y4+5y3−20y−16
8.1.10.13.
Answer.
  1. 1+15t+75t+125tt
  2. 1−9a+27a2−27a3
8.1.10.15.
Answer.
  1. 27a3−8b3
  2. 8a3+27b3
8.1.10.17.
Answer.
(a−2b)(a2+2ab+4b2)
8.1.10.19.
Answer.
(3a+4b)(9a2−12ab+16b2)
8.1.10.21.
Answer.
(4t3+w2)(16t6−4t3w2+w4)

Applications

8.1.10.23.
Answer.
  1. length: w+3; height: w−2
  2. w3+w2−6w
  3. 5w2+w−12
8.1.10.25.
Answer.
  1. 23πr3+πr2h
  2. V(r)=143πr3
8.1.10.27.
Answer.
  1. 0,9
  2. 0≤x≤9; R≥0 for these values
  3. cubic
  4. 283 points
  5. 36 points
  6. 3 ml or 8.2 ml
8.1.10.29.
Answer.
  1. 20 cm
  2. 100 cm
8.1.10.31.
Answer.
  1. 6+x+5x2
  2. 4−7x2−8x4

8.2 Algebraic Fractions
8.2.5 Problem Set 8.2

Warm Up

8.2.5.1.
Answer.
  1. −35, 37
  2. 3
  3. −1
8.2.5.3.
Answer.
  1. −43, 40399
  2. 1, −1
  3. 0
8.2.5.5.
Answer.
  1. 5x
  2. 12b7
8.2.5.7.
Answer.
  1. −85
  2. a9

Skills Practice

8.2.5.9.
Answer.
None are correct
8.2.5.11.
Answer.
  1. cannot be reduced
  2. 1
  3. cannot be reduced
  4. cannot be reduced
8.2.5.13.
Answer.
(b)
8.2.5.15.
Answer.
(a)
8.2.5.17.
Answer.
aa−3
8.2.5.19.
Answer.
1a+b
8.2.5.21.
Answer.
y+3xy−3x
8.2.5.23.
Answer.
y−2
8.2.5.25.
Answer.
−aa+1
8.2.5.27.
Answer.
4z2+6z+92z+3

Applications

8.2.5.29.
Answer.
  1. 30 min
  2. 50 min
  3. 50 min
  4. 6 mph
  5. The time increases. If the current is 10 mph, the team will not be able to row upstream
8.2.5.31.
Answer.
  1. 0≤p<100
  2. p 0 25 50 75 90 100
    C 0 120 360 1080 3240 −−
  3. curve
    60%
  4. p<80%
  5. p=100: The cost of extracting more ore grows without bound as the amount extracted approaches 100%.
8.2.5.33.
Answer.
  1. 2002x−1 square centimeters
  2. 8; If x=13, the area of the cross-section is 8 cm2.
8.2.5.35.
Answer.
  1. 4500+3000x; C(x)=6x+4500+3000x
  2. x 20 40 60 80 100
    C 4770 4815 5018 5130
  3. $4768.33
    rational function
  4. 22; 14>
  5. rational function with slant asymptote
    The graph of C approaches the line as an asymptote.

8.3 Operations on Algebraic Fractions
8.3.9 Problem Set 8.3

Warm Up

8.3.9.1.
Answer.
  1. 415
  2. 2z3w
8.3.9.3.
Answer.
  1. 28
  2. 28
8.3.9.5.
Answer.
  1. 16
  2. 16y2

Skills Practice

8.3.9.7.
Answer.
  1. −36a27
  2. 8b3b+3
  3. v21−v2
8.3.9.9.
Answer.
18x
8.3.9.11.
Answer.
a(2a−1)a+4
8.3.9.13.
Answer.
6x(x−2)(x−1)2(x2−8)(x2−2x+4)
8.3.9.15.
Answer.
9a314b3
8.3.9.17.
Answer.
3a2
8.3.9.19.
Answer.
x2y−1
8.3.9.21.
Answer.
(z+2)2z2(2z−1)
8.3.9.23.
Answer.
(x−y)(4x2+2xy+y2)
8.3.9.25.
Answer.
2x+y3x
8.3.9.27.
Answer.
z−3
8.3.9.29.
Answer.
10x+34x2
8.3.9.31.
Answer.
138a−4b
8.3.9.33.
Answer.
h2+2h−3h+2
8.3.9.35.
Answer.
6x−x2−4x(x−2)
8.3.9.37.
Answer.
196(p−2)
8.3.9.39.
Answer.
5k+1k(k−3)(k+1)
8.3.9.41.
Answer.
3y−3y2(y+1)(2y−1)
8.3.9.43.
Answer.
12xy2(x+y)2
8.3.9.45.
Answer.
x(x−1)3

Applications

8.3.9.47.
Answer.
4LRD2
8.3.9.49.
Answer.
2Lc+2LV2c3
8.3.9.51.
Answer.
3q8πR−a2q8πR3
8.3.9.53.
Answer.
−8t+14−3t
8.3.9.55.
Answer.
  1. x2
  2. 2x
  3. 12x
8.3.9.57.
Answer.
  1. 1a+b
  2. 34(a+b)
  3. 43(a+b)
8.3.9.59.
Answer.
−H+STRT
8.3.9.61.
Answer.
4L−R2C4L2C
8.3.9.63.
Answer.
2r22ra+a2a2
8.3.9.65.
Answer.
  1. 144x2−2x sq ft
  2. 48x−48x2−2xft
8.3.9.67.
Answer.
  1. 900400+w hr
  2. 900400−w hr
  3. Orville, by 1800w160,000−w2 hr

8.4 More Operations on Fractions
8.4.5 Problem Set 8.4

Warm Up

8.4.5.1.
Answer.
2x2+x−2x(x−1)
8.4.5.3.
Answer.
x+2x−1

Skills Practice

8.4.5.5.
Answer.
6y
8.4.5.7.
Answer.
516
8.4.5.9.
Answer.
710a+2
8.4.5.11.
Answer.
2x+1x
8.4.5.13.
Answer.
nqp+q
8.4.5.15.
Answer.
u−vxv
8.4.5.17.
Answer.
12x212−13x2
8.4.5.19.
Answer.
x−2+3y
8.4.5.21.
Answer.
2y+5+22y+1)
8.4.5.23.
Answer.
4z3−2z2+3z+1+22z+1

Applications

8.4.5.25.
Answer.
PQ― and overlineRS: ba; QR― and overlineSP: ba
8.4.5.27.
Answer.
  1. 1f=2q+60q2+60q
  2. f=q2+60q2q+60
8.4.5.29.
Answer.
n2−k2n2
8.4.5.31.
Answer.
2cdc2−u2
8.4.5.33.
Answer.
KLN(L−F)
8.4.5.35.
Answer.
1m+2h
8.4.5.37.
Answer.
x2+y2x2y2
8.4.5.39.
Answer.
b2−a2ab
8.4.5.41.
Answer.
yy−x
8.4.5.43.
Answer.
153
8.4.5.45.
Answer.
23+69
8.4.5.47.
Answer.
63+725

8.5 Equations with Fractions
8.5.8 Problem Set 8.5

Warm Up

8.5.8.1.
Answer.
−12
8.5.8.3.
Answer.
138
8.5.8.5.
Answer.
±158
8.5.8.7.
Answer.
18001849≈0.97

Skills Practice

8.5.8.9.
Answer.
−2, 1
8.5.8.11.
Answer.
−6
8.5.8.13.
Answer.
1
8.5.8.15.
Answer.
−145
8.5.8.17.
Answer.
We don’t multiply by the LCD in addition problems.
8.5.8.19.
Answer.
r=S−aa
8.5.8.21.
Answer.
x=a−ayb
8.5.8.23.
Answer.
R=Crr−C

Applications

8.5.8.25.
Answer.
2 mph
8.5.8.27.
Answer.
24 days
8.5.8.29.
Answer.
168=72p100−p; p=70%
8.5.8.31.
Answer.
  1. 0.268
  2. 44+x164+x
  3. 21
8.5.8.33.
Answer.
  1. t=144v−20
  2. t=144v+20
  3. (100,3)
  4. t=144v−20+t=144v+20=3
  5. 100 mph
8.5.8.35.
Answer.
2813 miles
8.5.8.37.
Answer.
  1. AE=1, DE=x−1, CD=1
  2. 1x=x−1x
  3. 1+52
8.5.8.39.
Answer.
Because x=1, dividing by x−1 in the fourth step is dividing by 0.
8.5.8.41.
Answer.
  1. two rational functions
  2. x=12

8.6 Chapter 8 Summary and Review
8.6.3 Chapter 8 Review Problems

8.6.3.1.

Answer.
2x3−11x2+19x−10

8.6.3.3.

Answer.
(2x−3z)(4x2+6xz+9z2)

8.6.3.5.

Answer.
  1. 16n3−12n2+13n
  2. 220
  3. 20

8.6.3.7.

Answer.
  1. V=πh34
  2. 2π cm3≈6.28 cm3; 16π cm3≈50.27 cm3
  3. cubic

8.6.3.9.

Answer.
  1. rational function
  2. 338
  3. Months 2 and 02
  4. During month 6. The number of members eventually decreases to zero.

8.6.3.11.

Answer.
  1. t1=90v−2
  2. t2=90v+2
  3. curve
  4. 90v−2+90v+2=24
  5. 8 mph

8.6.3.13.

Answer.
a2(a−1)

8.6.3.15.

Answer.
y2−2x2

8.6.3.17.

Answer.
a−32a+6)

8.6.3.19.

Answer.
10ab

8.6.3.21.

Answer.
6x2x+3

8.6.3.23.

Answer.
a2−2aa2+3a+2

8.6.3.25.

Answer.
12x−1

8.6.3.27.

Answer.
9x2−7+4x2−1x4

8.6.3.29.

Answer.
x2−2x−2−1x−2

8.6.3.31.

Answer.
2x

8.6.3.33.

Answer.
3x+12(x−3)(x+3)

8.6.3.35.

Answer.
2a2−a+1(a−3)(a−1)

8.6.3.37.

Answer.
15

8.6.3.39.

Answer.
xx+4

8.6.3.41.

Answer.
−2

8.6.3.43.

Answer.
No solution

8.6.3.45.

Answer.
n=CtC−V

8.6.3.47.

Answer.
q=prr−p

8.6.3.49.

Answer.
x3+yx3y

8.6.3.51.

Answer.
1−x2y2xy

8.6.3.53.

Answer.
−(x−y)2xy

9 Equations and Graphs
9.1 Properties of Lines
9.1.4 Problem Set 9.1

Warm Up

9.1.4.1.
Answer.
  1. A:negative; B: negative; C: positive; D: zero
  2. B, A, D, C
9.1.4.3.
Answer.
Number Negative
reciprocal		 Their
product
23 −32 −1
−52 25 −1
6 −16 −1
−4 14 −1
−1 1 −1

Skills Practice

9.1.4.5.
Answer.
  1. verticl line
  2. m is undefined; (4,0)
9.1.4.7.
Answer.
x=−5
9.1.4.9.
Answer.
y=6
9.1.4.11.
Answer.
parallel: a, g, h; perpendicular: c, f
9.1.4.13.
Answer.
  1. No
  2. 3 and 3.1; no
  3. 68. The two lines meet at (20,68).

Applications

9.1.4.15.
Answer.
b. mPQ=−52; mPR=25
9.1.4.17.
Answer.
  1. y=32x+52; the graph is below.
  2. 32
  3. parallel lines
  4. y=32x+72
9.1.4.19.
Answer.
  1. y=−2x−8
  2. y=12x−3
9.1.4.21.
Answer.
y=32x+152

9.2 The Distance and Midpoint Formulas
9.2.7 Problem Set 9.2

Warm Up

9.2.7.1.
Answer.
  1. False
  2. False
9.2.7.3.
Answer.
x −4 −3 −2 −1 0 1 2 3 4
y 0 ±7 ±12 ±15 ±4 ±15 ±12 ±7 0
circle of radius 4 centered at origin
9.2.7.5.
Answer.
5±332

Skills Practice

9.2.7.7.
Answer.
distance: 20; midpoint: (0,−2)
9.2.7.9.
Answer.
distance: 8; midpoint: (−2,−1)
9.2.7.11.
Answer.
center: (0,0); radius: 5
9.2.7.13.
Answer.
center: (−3,0); radius: 10

Applications

9.2.7.15.
Answer.
15+80+41≈30.3
9.2.7.17.
Answer.
50≈7.1
9.2.7.19.
Answer.
y=12x+54
9.2.7.21.
Answer.
  1. (220,−38.5)
  2. Both distances are 165.8 mi.
9.2.7.23.
Answer.
circle center at origin, radius 2
9.2.7.25.
Answer.
circle center at origin, radius 2
9.2.7.27.
Answer.
circle center at (0,-4) radius sqrt12
9.2.7.29.
Answer.
(x+4)2+y2=20; center: (−4,0); radius: 20
9.2.7.31.
Answer.
x2+(y−5)2=27; center: (0,5); radius: 27
9.2.7.33.
Answer.
(x−32)2+(y+4)2=294
9.2.7.35.
Answer.
(x+3)2+(y+1)2=1

9.3 Conic Sections: Ellipses
9.3.5 Problem Set 9.3

Warm Up

9.3.5.1.
Answer.
  1. x2+y2=r2
  2. x2r2+y2r2=1
  3. a=b=r
9.3.5.3.
Answer.
(−2,6)
9.3.5.5.
Answer.
x-intercepts: (−6,0), (4,0), vertex: (−1,252)

Skills Practice

9.3.5.7.
Answer.
circle
9.3.5.9.
Answer.
central ellipse
9.3.5.11.
Answer.
central ellipse
9.3.5.13.
Answer.
  1. x29+y24=1
  2. x 0 ±3 −2 ±332
    y ±2 0 ±252 1
9.3.5.15.
Answer.
  1. Radius 2
  2. (−1,±3)
9.3.5.17.
Answer.
  1. a=3, b=6
  2. None
9.3.5.19.
Answer.
  1. ellipse
  2. (−1,4), (7,4), (3,1), (3,7) (Others are possible.)
9.3.5.21.
Answer.
  1. x24+(y−2)29=1
  2. ellipse
9.3.5.23.
Answer.
  1. (x−3)21+(y+2)28=1
  2. ellipse
9.3.5.25.
Answer.
(x−1)29+(y−6)24=1
9.3.5.27.
Answer.
(x−3)225+(y−3)216=1

Applications

9.3.5.29.
Answer.
  1. x2102+y272=1
  2. 4.2 ft
9.3.5.31.
Answer.
  1. x2242+y282=1
  2. 10.29 ft

9.4 Conic Sections: Hyperbolas
9.4.6 Problem Set 9.4

Skills Practice

9.4.6.3.
Answer.
hyperbola, open up and down
9.4.6.5.
Answer.
hyperbola, open left and right
9.4.6.7.
Answer.
hyperbola, open left and right
9.4.6.9.
Answer.
  1. x29−y23=1
  2. x 0 ±352 5 ±154
    y undefined ±2 ±163 −3
9.4.6.11.
Answer.
  1. hyperbolas opening left and right
  2. hyperbolas opening left and right
9.4.6.13.
Answer.
  1. hyperbola opening up and down
  2. (−2,−2±6), (−2±5,1)
9.4.6.15.
Answer.
  1. hyperbola opening up and down
  2. (1−4±22), (−2,−8), (4,−8)
9.4.6.17.
Answer.
  1. hyperbola opening up and down
  2. (3±5), (3±22,3)
9.4.6.19.
Answer.
16x2−y2−192x−4y+556=0
9.4.6.21.
Answer.
x2−4y2+10x−16y−27=0
9.4.6.23.
Answer.
Parabola; vertex (0,2), opens downward, a=−12
9.4.6.25.
Answer.
Hyperbola; center (−18,−1), transverse axis vertical, a2=1565, b2=1516
9.4.6.27.
Answer.
Parabola; vertex (−4,2), opens upward, a=14

Applications

9.4.6.29.
Answer.
520 ft
9.4.6.31.
Answer.
472.5 ft

9.5 Nonlinear Systems
9.5.4 Problem Set 9.5

Warm Up

9.5.4.1.
Answer.
(1,−2)
9.5.4.3.
Answer.
x=3,16

Skills Practice

9.5.4.5.
Answer.
(−1,12),(4,7)
9.5.4.7.
Answer.
No solution
9.5.4.9.
Answer.
(1,4)
9.5.4.11.
Answer.
(2,2), (−2,−2)
9.5.4.13.
Answer.
(2,−1), (−2,1), (1,−2), (−1,2)
9.5.4.15.
Answer.
(±2,−±5)
9.5.4.17.
Answer.
(±6,−±2)
9.5.4.19.
Answer.
(0,4), (−2,0)

Applications

9.5.4.21.
Answer.
12 ft by 18 ft
9.5.4.23.
Answer.
P=6 lb per sq in; V=5 cu. in.
9.5.4.25.
Answer.
  1. grid
  2. 1000 or 5000
  3. 2000 or 4000
  4. harvst 1800; stable population 3000
  5. extinction
9.5.4.27.
Answer.
  1. (200, 2600),(1400, 18,200)
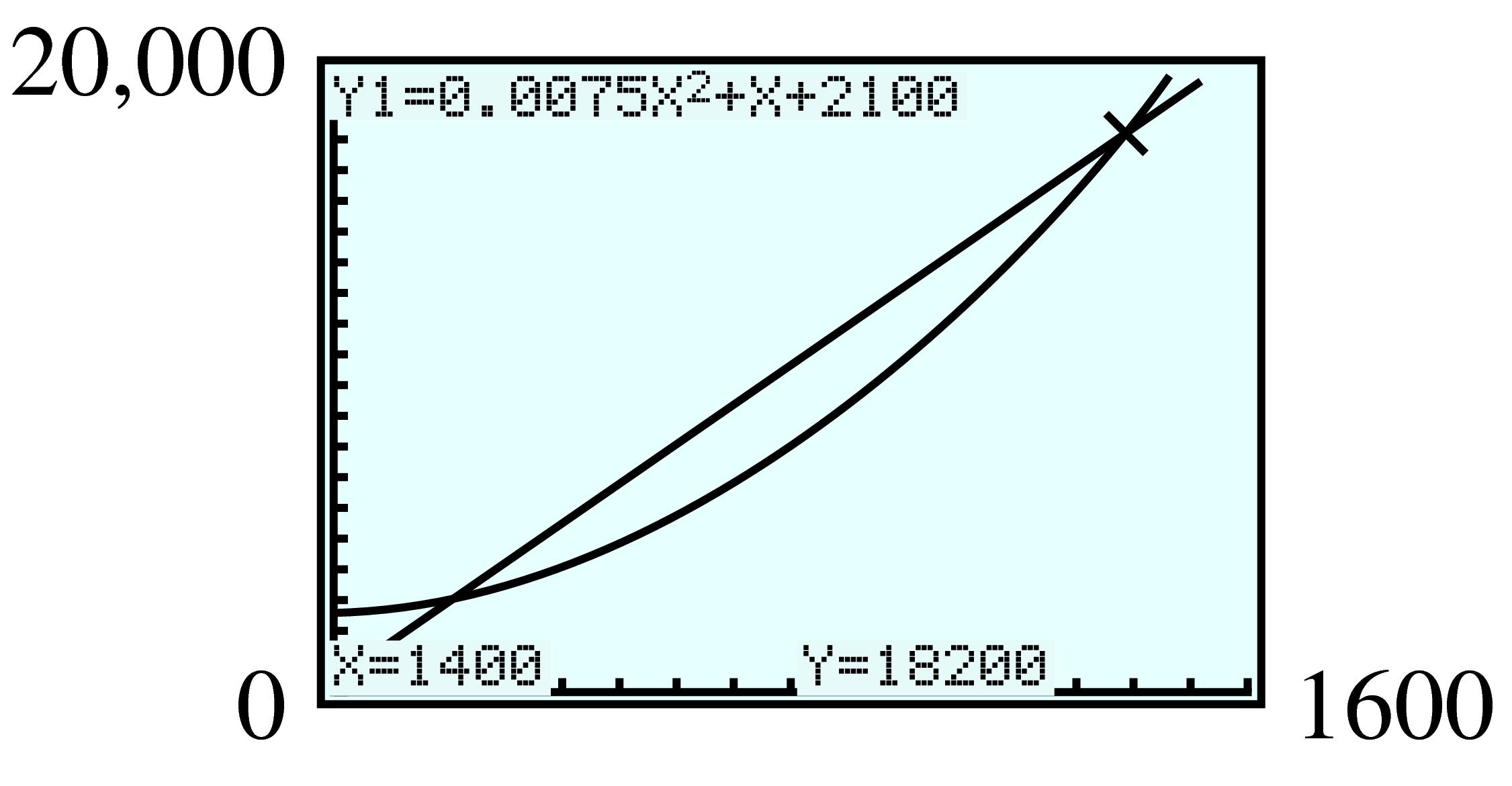
  2. parabola and line
  3. x=800

9.6 Chapter 9 Summary and Review
9.6.3 Chapter 9 Review Problems

9.6.3.1.

Answer.
parallel

9.6.3.2.

Answer.
perpendicular

9.6.3.3.

Answer.
y=−23x+143

9.6.3.4.

Answer.
y=32x+52

9.6.3.5.

Answer.
y=23x−263

9.6.3.6.

Answer.
y=32x−2

9.6.3.7.

Answer.
21.59; yes

9.6.3.8.

Answer.
10.8

9.6.3.9.

Answer.
circle

9.6.3.10.

Answer.
ellipse

9.6.3.11.

Answer.
ellipse

9.6.3.12.

Answer.
hyperbola

9.6.3.13.

Answer.
parabola

9.6.3.14.

Answer.
circle

9.6.3.15.

Answer.
ellipse

9.6.3.16.

Answer.
ellipse

9.6.3.17.

Answer.
hyperbola

9.6.3.18.

Answer.
parabola

9.6.3.19.

Answer.
  1. (x−2)2+(y+1)2=9
  2. Circle: center (2,−1), radius 3

9.6.3.20.

Answer.
  1. x2+(y−3)2=13
  2. Circle: center (0,3), radius 13

9.6.3.21.

Answer.
  1. (x−2)24+(y+2)216=1
  2. Ellipse: center (2,−2), a=2, b=4

9.6.3.22.

Answer.
  1. (x+1)25+(y−2)28=1
  2. Ellipse: center (−1,2), a=5, b=8

9.6.3.23.

Answer.
  1. y+10=(x−4)2
  2. Parabola: vertex (4,10), opens upward, a=1

9.6.3.24.

Answer.
  1. x−2=−14(y+3)2
  2. Parabola: vertex (2,−3), opens left, a=−14

9.6.3.25.

Answer.
  1. y+2=−(x−2)2
  2. Parabola: vertex (2,−2), opens downward, a=−1

9.6.3.26.

Answer.
  1. x+32=12(y−1)2
  2. Parabola: vertex (−32,1), opens right, a=12

9.6.3.27.

Answer.
  1. (y−4)26−(x+2)24=1
  2. Hyperbola: center (−2,4), transverse axis vertical, a=2, b=6

9.6.3.28.

Answer.
  1. (x−4)24−(y+3)29=1
  2. Hyperbola: center (4,−3), transverse axis horizontal, a=2, b=3

9.6.3.29.

Answer.
  1. x25−(y−3)210=1
  2. Hyperbola: center (0,3), transverse axis horizontal, a=5, b=10

9.6.3.30.

Answer.
  1. y23−(x−4)212=1
  2. Hyperbola: center (4,0), transverse axis vertical, a=23, b=310

9.6.3.31.

Answer.
  1. x225+y264=1
  2. ±245

9.6.3.32.

Answer.
  1. x2169+y281=1
  2. ±4513

9.6.3.33.

Answer.
(x+4)2+(y−3)2=20

9.6.3.34.

Answer.
(x+2)2+(y−4)2=13

9.6.3.35.

Answer.
(x+1)216+(y−4)24=1

9.6.3.36.

Answer.
(x−3)24+(y−1)225=1

9.6.3.37.

Answer.
(x−2)216−(y+3)29=1

9.6.3.38.

Answer.
(x+3)2−(y−1)29=1

9.6.3.39.

Answer.
(±2,±3)

9.6.3.40.

Answer.
(±3,±4)

9.6.3.41.

Answer.
(1,−2), (−1,2), (23,−13), (−23,13)

9.6.3.42.

Answer.
(342,342), (−342,−342)

9.6.3.43.

Answer.
Moia: 45 mph, Fran: 50 mph

9.6.3.44.

Answer.
12 in by 1 in

9.6.3.45.

Answer.
7 cm by 10 cm

9.6.3.46.

Answer.
7 ft by 2 ft

9.6.3.47.

Answer.
Morning train: 20 mph, evening train: 30 mph

9.6.3.48.

Answer.
Amount: $800, rate: 4%

10 Logarithmic Functions
10.1 Logarithmic Functions
10.1.7 Problem Set 10.1

Warm Up

10.1.7.1.
Answer.
9y=729
10.1.7.3.
Answer.
10−4.5=C
10.1.7.5.
Answer.
logb⁡(14)
10.1.7.7.
Answer.
log10⁡(xyz3)

Skills Practice

10.1.7.9.
Answer.
3 to x and log base 3
10.1.7.11.
Answer.
  1. 15.6144
  2. 0.4186
10.1.7.13.
Answer.
−1.58×10−5
10.1.7.15.
Answer.
  1. 25.70
  2. 3.31
10.1.7.17.
Answer.
  1. 12
  2. 01
10.1.7.19.
Answer.
  1. 81
  2. 4
  3. 1.8
  4. a
10.1.7.21.
Answer.
  1. IV
  2. V
  3. I
  4. II
  5. III
  6. VI
10.1.7.23.
Answer.
  1. 10,000
  2. 100,000,000
10.1.7.25.
Answer.
4
10.1.7.27.
Answer.
3

Applications

10.1.7.29.
Answer.
  1. data points and log curve
  2. The graph resembles a logarithmic function. The function is close to the points but appears too steep at first and not steep enough after n=15. Overall, it is a good fit.
  3. f grows (more and more slowly) without bound. f will eventually exceed 100 per cent, but no one can forget more than 100% of what is learned.
10.1.7.31.
Answer.
1962

10.2 Logarithmic Scales
10.2.7 Problem Set 10.2

Warm Up

10.2.7.1.
Answer.
  1. 0 and 1
  2. 2 and 3
  3. −1 and 0
  4. 6 and 7
10.2.7.3.
Answer.
  1. 3981.1
  2. 5.01
  3. 0.00079
  4. 0.398

Skills Practice

10.2.7.5.
Answer.
  1. log scale with exponents shown
  2. log scale with exponents shown
10.2.7.7.
Answer.
logscale
10.2.7.9.
Answer.
1.58, 6.31, 15.8, 63.1
10.2.7.11.
Answer.
3.2
10.2.7.13.
Answer.
0.0126
10.2.7.15.
Answer.
100
10.2.7.17.
Answer.
6,309,573 watts per square meter

Applications

10.2.7.19.
Answer.
1, 80, 330, 1600, 7000, 4×107
10.2.7.21.
Answer.
Proxima Centauri: 15.5; Barnard: 13.2; Sirius: 1.4; Vega: 0.6; Arcturus: −0.4; Antares: −4.7; Betelgeuse: −7.2
10.2.7.23.
Answer.
pH on log scale
10.2.7.25.
Answer.
103.4≈2512
10.2.7.27.
Answer.
A: a≈45, p≈7.4%; B: a≈400, p≈15%; C: a≈6000, p≈50%; D: a≈13000, p≈45%
10.2.7.29.
Answer.
12.6
10.2.7.32.
Answer.
3160
10.2.7.33.
Answer.
≈25,000

10.3 The Natural Base
10.3.7 Homework 10.3

Skills Practice

10.3.7.1.
Answer.
x −10 −5 0 5 10 15 20
f(x) 0.135 0.368 1 2.718 7.389 20.086 54.598
growth
10.3.7.3.
Answer.
x −10 −5 0 5 10 15 20
f(x) 20.086 4.482 1 0.223 0.05 0.011 0.00248
decay
10.3.7.5.
Answer.
  1. 2
  2. 5t
  3. 1x
  4. 12
10.3.7.7.
Answer.
  1. 0.64
  2. 3.81
  3. −1.20
10.3.7.9.
Answer.
  1. 4.14
  2. 1.88
  3. 0.07
10.3.7.11.
Answer.
P(t)=20(e0.4)t≈20⋅1.492t; increasing; initial value 20
10.3.7.13.
Answer.
P(t)=6500(e−2.5)t≈6500⋅0.082t; decreasing; initial value 6500
10.3.7.15.
Answer.
  1. x 0 0.5 1 1.5 2 2.5
    ex 1 1.6487 2.7183 4.4817 7.3891 12.1825
  2. Each ratio is e0.5≈1.6487: Increasing x-values by a constant Δx=0.5 corresponds to multiplying the y-values of the exponential function by a constant factor of eΔx.
10.3.7.17.
Answer.
  1. x 0 0.6931 1.3863 2.0794 2.7726 3.4657 4.1589
    ex 1 2 4 8 16 32 64
  2. Each difference in x-values is approximately ln⁡2≈0.6931: Increasing x-values by a constant Δx=ln⁡2 corresponds to multiplying the y-values of the exponential function by a constant factor of eΔx=eln⁡2=2. That is, each function value is approximately equal to double the previous one.
10.3.7.19.
Answer.
0.8277
10.3.7.21.
Answer.
−2.9720
10.3.7.23.
Answer.
1.6451
10.3.7.25.
Answer.
−3.0713
10.3.7.27.
Answer.
t=1kln⁡(y)
10.3.7.29.
Answer.
t=ln⁡(kk−y)
10.3.7.31.
Answer.
k=eT/T0−10
10.3.7.33.
Answer.
  1. n 0.39 3.9 39 390
    ln⁡(n) −0.942 1.361 3.664 5.966
  2. Each difference in function values is approximately ln⁡10≈2.303: Multiplying x-values by a constant factor of 10 corresponds to adding a constant value of ln⁡10 to the y-values of the natural log function.
10.3.7.35.
Answer.
  1. n 2 4 8 16
    ln⁡n 0.693 1.386 2.079 2.773
  2. Each quotient equals k, where n=2k. Because ln⁡(n)=ln⁡(2k)=k⋅ln⁡(2), k=ln⁡(n)ln⁡(2).
10.3.7.37.
Answer.
  1. N(t)=100e(ln⁡(2))t≈100e0.6931t
  2. growth
10.3.7.39.
Answer.
  1. N(t)=1200e(ln⁡(0.6))t≈1200e−0.5108t
  2. decay
10.3.7.41.
Answer.
  1. N(t)=10e(ln⁡(1.15))t≈10e0.1398t
  2. growth

Applications

10.3.7.43.
Answer.
  1. N(t)=6000e0.04t
  2. t 0 5 10 15 20 25 30
    N(t) 6000 7328 8951 10,933 13,353 16,310 19,921
  3. growth
  4. 15,670
  5. 70.3 hrs
10.3.7.45.
Answer.
  1. decay
  2. 941.8 lumens
  3. 2.2 cm
10.3.7.47.
Answer.
  1. 20,000
  2. (35,00020,000)1/10≈e0.056
  3. P(t)=20,000e0.056t
  4. 107,188
10.3.7.49.
Answer.
  1. (385500)1/2≈e−0.1307
  2. N(t)=500e−0.1307t
  3. 135.3 mg
10.3.7.51.
Answer.
  1. A(t)=500e0.095t
  2. 7.3 years
  3. 7.3 years
d–e
growth with marked doubling time
10.3.7.53.
Answer.
  1. 6 hours
  2. 6 hours
  3. decay with marked half-life
10.3.7.55.
Answer.
  1. 12N0, 14N0, 116N0
  2. decay
  3. N(t)=N0e−0.0866t
10.3.7.57.
Answer.
  1. decay fit on data
    y=116(0.975)t
  2. decay fit on data
    y=116(0.975)t
  3. G(t)=116e−0.025t
  4. 28 minutes

10.4 Chapter 10 Summary and Review
10.4.3 Chapter 10 Review Problems

10.4.3.5.

Answer.
−1

10.4.3.7.

Answer.
12

10.4.3.9.

Answer.
4

10.4.3.11.

Answer.
−158

10.4.3.13.

Answer.
94

10.4.3.15.

Answer.
3

10.4.3.17.

Answer.
x≈1.548

10.4.3.19.

Answer.
x≈411.58

10.4.3.21.

Answer.
x≈2.286

10.4.3.23.

Answer.
x

10.4.3.25.

Answer.
k−3

10.4.3.27.

Answer.
  1. P=7,894,862e−0.011t
  2. 1.095%

10.4.3.29.

Answer.
  1. $1419.07
  2. 13.9 years
  3. t=20ln⁡(A1000)

10.4.3.31.

Answer.
t=−1kln⁡(y−612)

10.4.3.33.

Answer.
M=NQt

10.4.3.35.

Answer.
P(t)=750(1.3771)t

10.4.3.37.

Answer.
N(t)=600e−0.9163t

10.4.3.39.

Answer.
log scale

10.4.3.41.

Answer.
Order 3: 17,000; Order 4: 5000; Order 8: 40; Order 9: 11
🔗
PrevTopNext